Article contents
Correspondences, von Neumann Algebras and Holomorphic L2 Torsion
Published online by Cambridge University Press: 20 November 2018
Abstract
Given a holomorphic Hilbertian bundle on a compact complex manifold, we introduce the notion of holomorphic 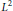 ${{L}^{2}}$ torsion, which lies in the determinant line of the twisted
${{L}^{2}}$ torsion, which lies in the determinant line of the twisted 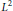 ${{L}^{2}}$ Dolbeault cohomology and represents a volume element there. Here we utilise the theory of determinant lines of Hilbertian modules over finite von Neumann algebras as developed in
${{L}^{2}}$ Dolbeault cohomology and represents a volume element there. Here we utilise the theory of determinant lines of Hilbertian modules over finite von Neumann algebras as developed in 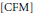 $[\text{CFM}]$. This specialises to the Ray-Singer-Quillen holomorphic torsion in the finite dimensional case. We compute a metric variation formula for the holomorphic
$[\text{CFM}]$. This specialises to the Ray-Singer-Quillen holomorphic torsion in the finite dimensional case. We compute a metric variation formula for the holomorphic 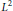 ${{L}^{2}}$ torsion, which shows that it is not in general independent of the choice of Hermitian metrics on the complex manifold and on the holomorphic Hilbertian bundle, which are needed to define it. We therefore initiate the theory of correspondences of determinant lines, that enables us to define a relative holomorphic
${{L}^{2}}$ torsion, which shows that it is not in general independent of the choice of Hermitian metrics on the complex manifold and on the holomorphic Hilbertian bundle, which are needed to define it. We therefore initiate the theory of correspondences of determinant lines, that enables us to define a relative holomorphic 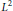 ${{L}^{2}}$ torsion for a pair of flat Hilbertian bundles, which we prove is independent of the choice of Hermitian metrics on the complex manifold and on the flat Hilbertian bundles.
${{L}^{2}}$ torsion for a pair of flat Hilbertian bundles, which we prove is independent of the choice of Hermitian metrics on the complex manifold and on the flat Hilbertian bundles.
Keywords
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2000
References
- 1
- Cited by


