Hostname: page-component-78c5997874-j824f Total loading time: 0 Render date: 2024-11-16T01:02:32.454Z Has data issue: false hasContentIssue false
  • English
  • Français

Covering Classes of Residues

Published online by Cambridge University Press:  20 November 2018

James H. Jordan
James H. Jordan*
Affiliation:
Washington State University, Pullman, Wash.
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

A set of ordered pairs of integers {(ai, mi)} is said to cover the integers if each integer x satisfies the congruence x ≡ ai (mod mi) for some i. We may assume that the mi are positive. Trivially {(0, 1)} covers, as does {(0, m), (1, m), (2, m), … , (m — 1, m)}. In order to arrive at some non-trivial problems concerning covers, the following definition is given: A finite set of ordered pairs of integers 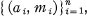with mi > 1 and mi ≠ mj if i ≠ j, is called a covering class of residues if every integer x satisfies the congruence x ≡ ai (mod mi) for some i.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 19 , 1967 , pp. 514 - 519
Copyright
Copyright © Canadian Mathematical Society 1967

References

1. Davenport, H., The higher arithmetic (New York, 1960). p. 57.Google Scholar
2. Erdös, P., On a problem concerning congruence systems, Mat. Lapok., 3 (1952), 122–128.Google Scholar
3. Erdös, P., Proceedings of the 1963 Number Theory Conference, University of Colorado, Proposed Problem No. 28.Google Scholar
4. Jordan, J. H. and Potratz, C. J., Complete residue systems in the Gaussian integers, Math. Mag., 38 (1965), 1–12.Google Scholar
5. Selfridge, J. L., Proceedings of the 1963 Number Theory Conference, University of Colorado, Proposed Problem No. 28.Google Scholar
6. Stein, S. K., Brief notes on unions of arithmetic progressions, Math. Dept., University of California at Davis.Google Scholar
7. Swift, J. D., Sets of covering congruences, Bull. Amer. Math. Soc., 60 (1954), 390.Google Scholar