Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Hartley, Richard
1980.
On the classification of three-braid links.
Abhandlungen aus dem Mathematischen Seminar der Universität Hamburg,
Vol. 50,
Issue. 1,
p.
108.
Mayberry, John P.
and
Murasugi, Kunio
1982.
Torsion-groups of abelian coverings of links.
Transactions of the American Mathematical Society,
Vol. 271,
Issue. 1,
p.
143.
Hartley, Richard
1983.
Identifying non-invertible knots.
Topology,
Vol. 22,
Issue. 2,
p.
137.
Murasugi, K.
1985.
On the height of 2-component links.
Topology and its Applications,
Vol. 19,
Issue. 3,
p.
227.
Murasugi, Kunio
1987.
Covering linkage invariants in abelian coverings of links.
Topology and its Applications,
Vol. 25,
Issue. 1,
p.
25.
Burde, Gerhard
1993.
Topics in Knot Theory.
p.
25.
Li, Gui-Song
1997.
Covering Sato-Levine invariants.
Transactions of the American Mathematical Society,
Vol. 349,
Issue. 12,
p.
5031.
HATAKENAKA, ERI
2010.
Invariants of 3-manifolds derived from covering presentations.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 149,
Issue. 2,
p.
263.
Kobayashi, Natsuka
Wada, Kodai
and
Yasuhara, Akira
2017.
Milnor invariants of covering links.
Topology and its Applications,
Vol. 224,
Issue. ,
p.
60.
Kjuchukova, Alexandra
2018.
Dihedral branched covers of four-manifolds.
Advances in Mathematics,
Vol. 332,
Issue. ,
p.
1.
Nagel, Matthias
Orson, Patrick
Park, JungHwan
and
Powell, Mark
2019.
Smooth and Topological Almost Concordance.
International Mathematics Research Notices,
Vol. 2019,
Issue. 23,
p.
7324.


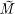 of
of 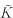 which may be a link of several components. The set of linking numbers between the various components of
which may be a link of several components. The set of linking numbers between the various components of 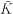 has long been recognised as a useful knot invariant. Bankwitz and Schumann used this invariant in considering dihedral coverings of Viergeflechte.
has long been recognised as a useful knot invariant. Bankwitz and Schumann used this invariant in considering dihedral coverings of Viergeflechte.