Hostname: page-component-78c5997874-t5tsf Total loading time: 0 Render date: 2024-11-13T06:38:59.620Z Has data issue: false hasContentIssue false
  • English
  • Français

Covering Maps and Periodic Functions on Higher Dimensional Sierpinski Gaskets

Published online by Cambridge University Press:  20 November 2018

Huo-Jun Ruan  and
Robert S. Strichartz
Huo-Jun Ruan
Affiliation:
(Ruan) Department of Mathematics, Zhejiang University, Hangzhou, 310027, China and Department of Mathematics, Cornell University, Ithaca, New York 14853, U.S.A., e-mail: ruanhj@zju.edu.cn
Robert S. Strichartz
Affiliation:
(Strichartz) Department of Mathematics, Cornell University, Ithaca, New York 14853, U.S.A., e-mail: str@math.cornell.edu
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We construct covering maps from infinite blowups of the $n$-dimensional Sierpinski gasket 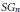$S{{G}_{n}}$ to certain compact fractafolds based on 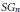$S{{G}_{n}}$. These maps are fractal analogs of the usual covering maps fromthe line to the circle. The construction extends work of the second author in the case 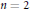$n=2$, but a differentmethod of proof is needed, which amounts to solving a Sudoku-type puzzle. We can use the covering maps to define the notion of periodic function on the blowups. We give a characterization of these periodic functions and describe the analog of Fourier series expansions. We study covering maps onto quotient fractalfolds. Finally, we show that such covering maps fail to exist for many other highly symmetric fractals.

Keywords

28A80
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 61 , Issue 5 , 01 October 2009 , pp. 1151 - 1181
Copyright
Copyright © Canadian Mathematical Society 2009

References

[Arm] Armstong, M. A., Groups and symmetry. Undergraduate Texts in Mathematics, Springer-Verlag, New York, 1988.Google Scholar
[FS] Fukushima, M. and Shima, T., On a spectral analysis for the Sierpinski gasket. Potential Anal. 1(1992), no. 1, 1–35.Google Scholar
[GS] Grigorchuk, R. and Z. Šunik, Asymptotic aspects of Schreier graphs and Hanoi Towers groups. C. R. Math. Acad. Sci. Paris 342(2006), no. 8, 545–550.Google Scholar
[Ki] Kigami, J., Analysis on fractals. Cambridge Tracts in Mathematics 143, Cambridge University Press, Cambridge, 2001.Google Scholar
[N] Nekrashevych, V., Self-Similar Groups. Mathematical Surveys and Monographs 117, American Mathematical Society, Providence, RI, 2005.Google Scholar
[Pa] Passman, D., Permutation groups. Benjamin, W. A., New York, 1968.Google Scholar
[Shi] Shirai, T., The spectrum of infinite regular line graphs. Trans. Amer. Math. Soc. 352(2000), no. 1, 115–132.Google Scholar
[S1] Strichartz, R. S., Fractals in the large. Canad. J. Math. 50(1998), no. 3, 638–657.Google Scholar
[S2] Strichartz, R. S.. Fractafolds based on the Sierpinski gasket and their spectra. Trans. Amer. Math. Soc. 355(2003), no. 10, 4019–4043.Google Scholar
[S3] Strichartz, R. S., Differential equations on fractals. A tutorial. Princeton University Press, Princeton, NJ, 2006.Google Scholar
[S4] Strichartz, R. S., Periodic and almost periodic functions on infinite Sierpinski gaskets. Canad. J. Math. 61(2009), 1182–1200.Google Scholar
[T] Teplyaev, A., Spectral analysis on infinite Sierpinski gaskets. J. Funct. Anal. 159(1998), no. 2, 537–567.Google Scholar