Published online by Cambridge University Press: 20 November 2018
In a recent paper we studied systems of equations of the form
(1) 
(2) 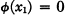
where as usual [a,b] = ab — ba and ϕ(λ) is a polynomial. Equations of this type have arisen in quantum mechanics. In our paper we gave a method of determining the matrix solutions of such equations. The starting point of our discussion was the observation that if the elements xi satisfy (1) then the elements xi, [xj,xk] satisfy the multiplication table of a certain basis of the Lie algebra 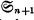 of skew symmetric (n + 1) ⨯ (n + 1) matrices. We proved that if (2) is imposed as an added condition, then the algebra generated by the
of skew symmetric (n + 1) ⨯ (n + 1) matrices. We proved that if (2) is imposed as an added condition, then the algebra generated by the 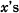 has a finite basis, and we obtained the structure of the most general associative algebra that is generated in this way.
has a finite basis, and we obtained the structure of the most general associative algebra that is generated in this way.