Hostname: page-component-78c5997874-dh8gc Total loading time: 0 Render date: 2024-11-10T16:11:40.789Z Has data issue: false hasContentIssue false
  • English
  • Français

Explicit Models for Threefolds Fibred by K3 Surfaces of Degree Two

Published online by Cambridge University Press:  20 November 2018

Alan Thompson
Alan Thompson*
Affiliation:
Department of Mathematical and Statistical Sciences, University of Alberta, Edmonton, AB, T6G 2G1, e-mail: amthomps@ualberta.ca
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We consider threefolds that admit a fibration by 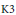$\text{K3}$ surfaces over a nonsingular curve, equipped with a divisorial sheaf that defines a polarization of degree two on the general fibre. Under certain assumptions on the threefold we show that its relative log canonical model exists and can be explicitly reconstructed from a small set of data determined by the original fibration. Finally, we prove a converse to this statement: under certain assumptions, any such set of data determines a threefold that arises as the relative log canonical model of a threefold admitting a fibration by 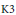$\text{K3}$ surfaces of degree two.

Keywords

14J3014D0614E3014J28threefoldfibrationK3 surface
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 65 , Issue 4 , 01 August 2013 , pp. 905 - 926
Copyright
Copyright © Canadian Mathematical Society 2013

References

[1] Avram, A. C., Kreuzer, M., Mandelberg, M., and Skarke, H., Searching for K3 fibrations. Nuclear Phys. B 494(1997), no. 3, 567–589. http://dx.doi.org/10.1016/S0550-3213(97)00214-9 Google Scholar
[2] Catanese, F. and Pignatelli, R., Fibrations of low genus I. Ann. Sci. École Norm. Sup. (4) 39(2006), no. 6, 1011–1049.Google Scholar
[3] Fujino, O., Introduction to the log minimal model program for log canonical pairs. arxiv:0907.1506. Google Scholar
[4] Hartshorne, R., Algebraic geometry. Graduate Texts in Mathematics, 52, Springer-Verlag, New York-Heidelberg, 1977.Google Scholar
[5] Hartshorne, R., Stable reflexive sheaves. Math. Ann. 254(1980), no. 2, 121–176. http://dx.doi.org/10.1007/BF01467074 Google Scholar
[6] Horikawa, E., On algebraic surfaces with pencils of curves of genus 2. In: Complex analysis and algebraic geometry, Iwanami Shoten, Tokyo, 1977, pp. 79–90.Google Scholar
[7] Hosono, S., Lian, B. H., and Yau, S.-T., Calabi-Yau varieties and pencils of K3 surfaces. arxiv:alg-geom/9603020. Google Scholar
[8] Hunt, B. and Schimmrigk, R., K3-fibered Calabi-Yau threefolds I. The twist map. Internat. J. Math. 10(1999), no. 7, 833–869. http://dx.doi.org/10.1142/S0129167X99000367 Google Scholar
[9] Kawamata, Y., Matsuda, K., and Matsuki, K., Introduction to the minimal model problem. In: Algebraic geometry, Sendai, 1985, Adv. Stud. Pure Math., 10, North-Holland, Amsterdam, 1987, pp. 283–360.Google Scholar
[10] Kollár, J. and Mori, S., Birational geometry of algebraic varieties. Cambridge Tracts in Mathematics, 134, Cambridge University Press, Cambridge, 1998.Google Scholar
[11] Mayer, A. L., Families of K – 3 surfaces. Nagoya Math. J. 48(1972), 1–17.Google Scholar
[12] Mendes-Lopes, M., The relative canonical algebra for genus three fibrations. Ph.D. thesis, University of Warwick, 1989.Google Scholar
[13] Mullet, J. P., Toric Calabi-Yau hypersurfaces fibered by weighted K3 hypersurfaces. Comm. Anal. Geom. 17(2009), no. 1, 107–138.Google Scholar
[14] Reid, M., Canonical 3-folds. In: Journées de Géométrie Algébrique d'Angers, Juillet 1979, Sijthoff & Noordhoff, Alphen aan den Rijn, 1980, pp. 273–310.Google Scholar
[15] Thompson, A., Degenerations of K3 surfaces of degree two arxiv:1010.5906. Google Scholar
[16] Thompson, A., Models for threefolds fibred by K3 surfaces of degree two Ph.D. thesis, University of Oxford, 2011.Google Scholar