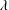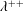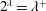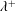Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Rinot, Assaf
and
Shalev, Roy
2023.
A guessing principle from a Souslin tree, with applications to topology.
Topology and its Applications,
Vol. 323,
Issue. ,
p.
108296.
Rinot, Assaf
Yadai, Shira
and
You, Zhixing
2024.
Full Souslin trees at small cardinals.
Journal of the London Mathematical Society,
Vol. 110,
Issue. 1,