No CrossRef data available.
Published online by Cambridge University Press: 20 November 2018
We write formally  (C, p) indicating that the integral is summable (C, p), i.e.,
(C, p) indicating that the integral is summable (C, p), i.e.,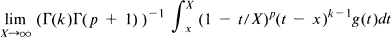 if this limit exists. We note here that all integrals over a finite range are taken in the Lebesgue sense, and all inversions of such iterated integrals are justifiable by Fubini's Theorem.
if this limit exists. We note here that all integrals over a finite range are taken in the Lebesgue sense, and all inversions of such iterated integrals are justifiable by Fubini's Theorem.