A Further Extension of Cayley's Parameterization

Extract

In a recent paper (3)* the following theorem was proved for real matrices.

Theorem 1. If A is a symmetric matrix and Q is a skew-symmetric matrix such that A + Q is non-singular, then

1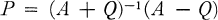

is a cogredient automorph (c.a.) of A whose determinant is + 1 and having theproperty that A and I + P span the same row space.

Conversely, if P is a c.a. of A whose determinant is + 1 and if P has theproperty that I + P and A span the same row space, then there exists a skew symmetricmatrix Q such that P is given by equation (1).

Theorem 1 reduces to the well-known Cayley parameterization in the case where A is non-singular. A similar and somewhat simpler result (Theorem 4) was given for the case when the underlying field is the complex field. It was also shown that the second part of the theorem (in either form) is false when the characteristic of the underlying field is 2. The purpose of this paper is to simplify the proof of Theorem 1 and at the same time, to extend these results to matrices over an arbitrary field of characteristic ≠ 2.