Published online by Cambridge University Press: 20 November 2018
We consider the positive zeros j″vk, k = 1, 2,…, of the second derivative of the Bessel function Jν(x). We are interested first in how many zeros there are on the interval (0,jν1), where jν1 is the smallest positive zero of Jν(x). We show that there exists a number ƛ = —0.19937078… such that 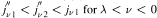 and
and 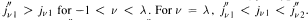 . Moreover, j″v1 decreases to 0 and j″ν2 increases to j″01 as ν increases from ƛ to 0. Further, j″vk increases in —1 < ν< ∞, for k = 3,4,… Monotonicity properties are established also for ordinates, and the slopes at the ordinates, of the points of inflection when — 1 < ν < 0.
. Moreover, j″v1 decreases to 0 and j″ν2 increases to j″01 as ν increases from ƛ to 0. Further, j″vk increases in —1 < ν< ∞, for k = 3,4,… Monotonicity properties are established also for ordinates, and the slopes at the ordinates, of the points of inflection when — 1 < ν < 0.