No CrossRef data available.
Published online by Cambridge University Press: 20 November 2018
Gelbart and Piatetskii-Shapiro constructed various integral representations of Rankin–Selberg type for groups  $G\,\times \,G{{L}_{n}}$, where
$G\,\times \,G{{L}_{n}}$, where 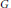 $G$ is of split rank
$G$ is of split rank  $n$. Here we show that their method can equally well be applied to the product
$n$. Here we show that their method can equally well be applied to the product 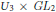 ${{U}_{3}}\,\times \,G{{L}_{2}}$, where
${{U}_{3}}\,\times \,G{{L}_{2}}$, where  ${{U}_{3}}$ denotes the quasisplit unitary group in three variables. As an application, we describe which cuspidal automorphic representations of
${{U}_{3}}$ denotes the quasisplit unitary group in three variables. As an application, we describe which cuspidal automorphic representations of  ${{U}_{3}}$ occur in the Siegel induced residual spectrum of the quasisplit
${{U}_{3}}$ occur in the Siegel induced residual spectrum of the quasisplit 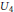 ${{U}_{4}}$.
${{U}_{4}}$.