K-Theory of Non-Commutative Spheres Arising from the Fourier Automorphism

Abstract

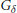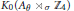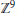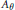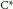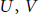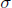For a dense ${{G}_{\delta }}$ set of real parameters $\theta $ in [0, 1] (containing the rationals) it is shown that the group ${{K}_{0}}({{A}_{\theta }}\,{{\rtimes }_{\sigma }}\,{{\mathbb{Z}}_{4}})$ is isomorphic to ${{\mathbb{Z}}^{9}}$, where ${{A}_{\theta }}$ is the rotation ${{\text{C}}^{*}}$-algebra generated by unitaries $U,\,V$ satisfying $VU\,=\,{{e}^{2\pi i\theta }}UV$ and $\sigma $ is the Fourier automorphism of 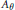${{A}_{\theta }}$ defined by $\sigma (U)\,=\,V,\,\sigma (V)\,=\,{{U}^{-1}}$. More precisely, an explicit basis for 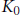${{K}_{0}}$ consisting of nine canonical modules is given. (A slight generalization of this result is also obtained for certain separable continuous fields of unital 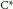${{\text{C}}^{*}}$-algebras over [0, 1].) The Connes Chern character 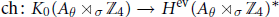$\text{ch:}\,{{K}_{0}}({{A}_{\theta }}\,{{\rtimes }_{\sigma }}\,{{\mathbb{Z}}_{4}})\,\to \,{{H}^{\text{ev}}}{{({{A}_{\theta \,}}{{\rtimes }_{\sigma }}\,{{\mathbb{Z}}_{4}})}^{*}}$ is shown to be injective for a dense 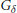${{G}_{\delta }}$ set of parameters $\theta $. The main computational tool in this paper is a group homomorphism $\mathbf{T}\,\text{:}\,{{K}_{0}}({{A}_{\theta }}\,{{\rtimes }_{\sigma }}\,{{\mathbb{Z}}_{4}})\,\to \,{{\mathbb{R}}^{8}}\,\times \,\mathbb{Z}$ obtained from the Connes Chern character by restricting the functionals in its codomain to a certain nine-dimensional subspace of 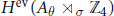${{H}^{\text{ev}}}({{A}_{\theta }}\,{{\rtimes }_{\sigma }}\,{{\mathbb{Z}}_{4}})$. The range of $\mathbf{T}$ is fully determined for each $\theta $. (We conjecture that this subspace is all of 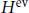${{H}^{\text{ev}}}$.)