Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-15T03:29:40.980Z Has data issue: false hasContentIssue false
  • English
  • Français

Mercerian Conditions for the Method (F, dn)

Published online by Cambridge University Press:  20 November 2018

H. B. Skerry
H. B. Skerry*
Affiliation:
Lehigh University, Bethlehem, Pennsylvania
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

This paper sets forth conditions sufficient that the generalized Lototsky method (F, dn) be regular and Mercerian. If the dn's are real and of constant sign, then the conditions are also necessary. Moreover, it follows that if f is a polynomial, then under the same conditions the method (f,dn) is equivalent to the Sonnenschein method generated by f . Various related results are also given.

Definition 2.1. Let f be a nonconstant function holomorphic on the closed unit disk and let 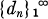be a complex sequence with f (l) + dn ≠ 0. Suppose

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 23 , Issue 6 , December 1971 , pp. 1078 - 1085
Copyright
Copyright © Canadian Mathematical Society 1971

References

1. Agnew, R. P., Comparison of products of methods of summability, Trans. Amer. Math. Soc. 43 (1938), 327–343.Google Scholar
2. Agnew, R. P., Equivalence of methods for evaluation of sequences, Proc. Amer. Math. Soc. 3 (1952), 550–556.Google Scholar
3. Clunie, J. and Vermes, P., Regular Sonnenschein type summability methods, Acad. Roy. Belg. Bull. CI. Sci. 45 (1959), 930–954.Google Scholar
4. Jakimovski, A., A generalization of the Lototsky method of summability, Michigan Math. J. 6 (1959), 277–290.Google Scholar
5. Jakimovski, A. and Skerry, H., Some regularity conditions for the (f, dn, Z\) summability method, Proc. Amer. Math. Soc. 24 (1970), 281–287.Google Scholar
6. Koch, C. F., Some remarks concerning (f, dn) and [F, dn] summability methods, Can. J. Math. 21 (1969), 1361–1365.Google Scholar
7. Lorentz, G. G., Bernstein polynomials (University of Toronto Press, Toronto, 1953).Google Scholar
8. Meir, A., On the [F, dn]-transformations of A. Jakimovski, Bull. Res. Council Israel Sect. F 10 (1962), 165–187.Google Scholar
9. Milne-Thomson, L. M., The calculus of finite differences (Macmillan, London, 1933).Google Scholar
10. Smith, G., On the (f, dn)-method of summability, Can. J. Math. 17 (1965), 506–526.Google Scholar
11. Wilansky, A. and Zeller, K., Abschnittsbeschrdnkte Matrix-transformationen; starke Limitierbarkeit, Math. Z. 64 (1956), 258–269.Google Scholar