Article contents
The Method of Upper and Lower Solutions for Some Nonlinear Boundary Value Problems in Unbounded Domains
Published online by Cambridge University Press: 20 November 2018
Extract
Let D be a bounded domain in the Euclidean space RN(N ≦ 2) and let 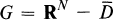 where
where 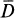 is the closure of D. We assume that the boundary ∂G of G is smooth. Consider the boundary value problem (abbreviated to BVP in the sequel).
is the closure of D. We assume that the boundary ∂G of G is smooth. Consider the boundary value problem (abbreviated to BVP in the sequel).
(1)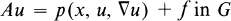
(1)
where A is a nonlinear elliptic differential operator in divergence form of Leray-Lions type, ∇u = grad u, f is a distribution on G and p(x, t, η) is a function defined on G × R × RN. Among other hypotheses we shall, roughly speaking, assume that p has completely unrelated growth rates in the first and the third variables. In this paper we prove the solvability of the BVP (1), (2) under the assumption that it has both an upper solution ψ and a lower solution φ with φ ≧ ψ.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1985
References
- 1
- Cited by


