Published online by Cambridge University Press: 20 November 2018
The non-archimedean power series spaces, 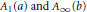 ${{A}_{1}}(a)\,\text{and}\,{{A}_{\infty }}(b)$, are the best known and most important examples of non-archimedean nuclear Fréchet spaces. We prove that the range of every continuous linear map from
${{A}_{1}}(a)\,\text{and}\,{{A}_{\infty }}(b)$, are the best known and most important examples of non-archimedean nuclear Fréchet spaces. We prove that the range of every continuous linear map from 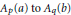 ${{A}_{p}}(a)\,\text{to}\,{{A}_{q}}(b)$ has a Schauder basis if either
${{A}_{p}}(a)\,\text{to}\,{{A}_{q}}(b)$ has a Schauder basis if either 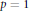 $p\,=\,1$ or
$p\,=\,1$ or  $p\,=\,\infty $ and the set
$p\,=\,\infty $ and the set 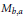 ${{M}_{b,a}}$ of all bounded limit points of the double sequence (
${{M}_{b,a}}$ of all bounded limit points of the double sequence (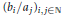 ${{({{b}_{i}}/{{a}_{j}})}_{i,j\in \mathbb{N}}}$ is bounded. It follows that every complemented subspace of a power series space
${{({{b}_{i}}/{{a}_{j}})}_{i,j\in \mathbb{N}}}$ is bounded. It follows that every complemented subspace of a power series space  ${{A}_{p}}(a)$ has a Schauder basis if either
${{A}_{p}}(a)$ has a Schauder basis if either 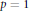 $p\,=\,1$ or
$p\,=\,1$ or  $p\,=\,\infty $ and the set
$p\,=\,\infty $ and the set 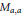 ${{M}_{a,a}}$ is bounded.
${{M}_{a,a}}$ is bounded.