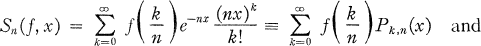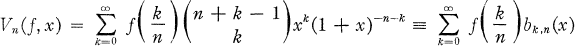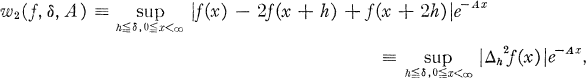Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Jayasri, C.
and
Sitaraman, Y.
1993.
On a Bernstein-type operator of Bleimann, Butzer and Hahn.
Journal of Computational and Applied Mathematics,
Vol. 47,
Issue. 2,
p.
267.
Guo, Feng
2003.
On convergence rate of approximation for two-dimensional Baskakov operators.
Analysis in Theory and Applications,
Vol. 19,
Issue. 3,
p.
273.
Cao, Feilong
Ding, Chunmei
and
Xu, Zongben
2005.
On multivariate Baskakov operator.
Journal of Mathematical Analysis and Applications,
Vol. 307,
Issue. 1,
p.
274.
Cao, Feilong
and
Ding, Chunmei
2008.
Lp approximation by multivariate Baskakov–Kantorovich operators.
Journal of Mathematical Analysis and Applications,
Vol. 348,
Issue. 2,
p.
856.
Doğru, Ogün
2008.
Direct and Inverse Theorems on Statistical Approximations by Positive Linear Operators.
Rocky Mountain Journal of Mathematics,
Vol. 38,
Issue. 6,
Wang, Jianjun
Guo, Haifeng
and
Jing, Jia
2013.
Estimation of Approximation with Jacobi Weights by Multivariate Baskakov Operator.
Journal of Function Spaces and Applications,
Vol. 2013,
Issue. ,
p.
1.
İzgi, Aydın
2013.
Approximation by a composition of Chlodowsky operators and Százs–Durrmeyer operators on weighted spaces.
LMS Journal of Computation and Mathematics,
Vol. 16,
Issue. ,
p.
388.
Atakut, Çiğdem
Serenbay, Sevilay
and
Büyükyazici, İbrahim
2013.
Approximation properties for generalized Baskakov-type operators.
Mathematical Sciences,
Vol. 7,
Issue. 1,
p.
21.
Gao, Yi
Wang, Wenshuai
and
Yue, Shigang
2015.
On the Rate of Convergence by Generalized Baskakov Operators.
Advances in Mathematical Physics,
Vol. 2015,
Issue. ,
p.
1.
Gupta, Vijay
and
Tachev, Gancho
2017.
Approximation with Positive Linear Operators and Linear Combinations.
Vol. 50,
Issue. ,
p.
89.
Erençin, Ayşegül
Olgun, Ali
and
Taşdelen, Fatma
2017.
Generalized Baskakov type operators.
Mathematica Slovaca,
Vol. 67,
Issue. 5,
p.
1269.
Gupta, Vijay
and
Tachev, Gancho
2017.
On Approximation Properties of Phillips Operators Preserving Exponential Functions.
Mediterranean Journal of Mathematics,
Vol. 14,
Issue. 4,
Gupta, Vijay
and
Agrawal, Deepika
2019.
Convergence by modified Post-Widder operators.
Revista de la Real Academia de Ciencias Exactas, Físicas y Naturales. Serie A. Matemáticas,
Vol. 113,
Issue. 2,
p.
1475.
Tachev, Gancho
Gupta, Vijay
and
Aral, Ali
2020.
Voronovskaja’s theorem for functions with exponential growth.
Georgian Mathematical Journal,
Vol. 27,
Issue. 3,
p.
459.
Gupta, Vijay
2020.
Approximation with certain exponential operators.
Revista de la Real Academia de Ciencias Exactas, Físicas y Naturales. Serie A. Matemáticas,
Vol. 114,
Issue. 2,
Gupta, Vijay
2020.
Convergence Estimates for Gamma Operator.
Bulletin of the Malaysian Mathematical Sciences Society,
Vol. 43,
Issue. 3,
p.
2065.
Mishra, Nav Shakti
and
Deo, Naokant
2021.
On the preservation of functions with exponential growth by modified Ismail–May operators.
Mathematical Methods in the Applied Sciences,
Vol. 44,
Issue. 11,
p.
9012.
Gupta, Vijay
and
Rassias, Michael Th.
2021.
Computation and Approximation.
p.
1.
Gupta, Vijay
Aral, Ali
and
Özsaraç, Firat
2022.
On semi-exponential Gauss–Weierstrass operators.
Analysis and Mathematical Physics,
Vol. 12,
Issue. 5,
Aslan, Reşat
and
Mursaleen, M.
2022.
Approximation by bivariate Chlodowsky type Szász–Durrmeyer operators and associated GBS operators on weighted spaces.
Journal of Inequalities and Applications,
Vol. 2022,
Issue. 1,