Hostname: page-component-78c5997874-t5tsf Total loading time: 0 Render date: 2024-11-11T06:06:23.844Z Has data issue: false hasContentIssue false
  • English
  • Français

On Multiple Mixed Interior and Boundary Peak Solutions for Some Singularly Perturbed Neumann Problems

Published online by Cambridge University Press:  20 November 2018

Changfeng Gui  and
Juncheng Wei
Changfeng Gui
Affiliation:
Department of Mathematics, University of British Columbia, Vancouver, British Columbia email: cgui@math.ubc.ca
Juncheng Wei
Affiliation:
Department of Mathematics, The Chinese University of Hong Kong, Shatin, Hong Kong email: wei@math.cuhk.edu.hk
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We consider the problem

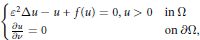$$\left\{ \begin{matrix} {{\varepsilon }^{2}}\Delta u-u+f(u)=0,u>0 & \text{in}\,\Omega \\ \frac{\partial u}{\partial v}=0\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, & \text{on}\,\partial \Omega , \\\end{matrix} \right.$$

where $\Omega $ is a bounded smooth domain in 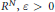${{R}^{N}},\varepsilon >0$ is a small parameter and $f$ is a superlinear, subcritical nonlinearity. It is known that this equation possesses multiple boundary spike solutions that concentrate, as $\varepsilon $ approaches zero, at multiple critical points of the mean curvature function 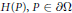$H(P),P\in \partial \Omega $. It is also proved that this equation has multiple interior spike solutions which concentrate, as $\varepsilon \to 0$, at sphere packing points in $\Omega $.

In this paper, we prove the existence of solutions with multiple spikes both on the boundary and in the interior. The main difficulty lies in the fact that the boundary spikes and the interior spikes usually have different scales of error estimation. We have to choose a special set of boundary spikes to match the scale of the interior spikes in a variational approach.

Keywords

35B4035B4535J40mixed multiple spikesnonlinear elliptic equations
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 52 , Issue 3 , 01 June 2000 , pp. 522 - 538
Copyright
Copyright © Canadian Mathematical Society 2000

References

[1] Adimurthi, G. Mancinni and Yadava, S. L., The role of mean curvature in a semilinear Neumann problem involving the critical Sobolev exponent. Comm. Partial Differential Equations 20(1995), 591–631.Google Scholar
[2] Adimurthi, F. Pacella and Yadava, S. L., Interaction between the geometry of the boundary and positive solutions of a semilinear Neumann problem with critical nonlinearity . J. Funct. Anal. 31(1993), 8–350.Google Scholar
[3] Adimurthi, F. Pacella and Yadava, S. L., Characterization of concentration points and L∞-estimates for solutions involving the critical Sobolev exponent. Integral Equation (1) 8(1995), 41–68.Google Scholar
[4] Bates, P. and Fusco, G., Equilibria with many nuclei for the Cahn-Hilliard equation. preprint.Google Scholar
[5] Bates, P., Dancer, E. N. and , Shi, Multi-spike stationary solutions of the Cahn-Hilliard equation in higherdimension and instability. preprint.Google Scholar
[6] Cerami, G. and Wei, J., Multiplicity of multiple interior spike solutions for some singularly perturbed Neumann problem. Internat.Math. Research Notes 12(1998), 601–626.Google Scholar
[7] del Pino, M., Felmer, P. and Wei, J., On the role of mean curvature in some singularly perturbed Neumann problems. SIAM J. Math. Anal., to appear.Google Scholar
[8] del Pino, M., Felmer, P. and Wei, J., On the role of the distance function for some singular perturbation problems. Comm. Partial Differential Equations, to appear.Google Scholar
[9] Dancer, E. N., A note on asymptotic uniqueness for some nonlinearities which change sign. RockyMountain J. Math., to appear.Google Scholar
[10] Dancer, E. N. and Yan, S., Multiple boundary peak solutions for a singularly perturbed Neumann problem. preprint.Google Scholar
[11] Floer, A. and Weinstein, A., Nonspreading wave packets for the cubic Schrödinger equation with a bounded potential. J. Funct. Anal. 69(1986), 397–408.Google Scholar
[12] Gidas, B., Ni, W.-M. and Nirenberg, L., Symmetry of positive solutions of nonlinear elliptic equations in Rn . Mathematical Analysis and Applications, Part A, Adv. Math. Suppl. Studies 7A, Academic Press, New York, 1981, 369–402.Google Scholar
[13] Gui, C., Multi-peak solutions for a semilinear Neumann problem. DukeMath. J. 84(1996), 739–769.Google Scholar
[14] Gui, C., Wei, J. and Winter, M., Multiple boundary peak solutions for some singularly perturbed Neumann problems. Ann. Inst. H. Poincaré Anal.Non Linéaire, to appear.Google Scholar
[15] Gui, C. and Ghoussoub, N., Multi-peak Solutions for a Semilinear Neumann Problem Involving the Critical Sobolev Exponent. Math. Z. 229(1998), 443–473.Google Scholar
[16] Gilbarg, D. and Trudinger, N. S., Elliptic Partial Differential Equations of Second Order. 2nd edition, Springer, Berlin, 1983.Google Scholar
[17] Gui, C. and Wei, J., Multiple Interior peak solutions for some singularly perturbed Neumann problems. J. Differential Equations 158(1999), 1–27.Google Scholar
[18] Lin, C., Ni, W.-M. and Takagi, I., Large amplitude stationary solutions to a chemotaxis systems. J. Differential Equations 72(1988), 1–27.Google Scholar
[19] Li, Y. Y., On a singularly perturbed equation with Neumann boundary condition. Comm. Partial Differential Equations 23(1998), 487–545.Google Scholar
[20] Ni, W.-M., Pan, X. and Takagi, I., Singular behavior of least-energy solutions of a semilinear Neumann problem involving critical Sobolev exponents. DukeMath. J. 67(1992), 1–20.Google Scholar
[21] Ni, W.-M. and Takagi, I., On the shape of least energy solution to a semilinear Neumann problem. Comm. Pure Appl. Math. 41(1991), 819–851.Google Scholar
[22] Ni, W.-M. and Takagi, I., Locating the peaks of least energy solutions to a semilinear Neumann problem. Duke Math. J. 70(1993), 247–281.Google Scholar
[23] Ni, W.-M. and Takagi, I., Point-condensation generated be a reaction-diffusion system in axially symmetric domains. Japan J. Industrial Appl. Math. 12(1995), 327–365.Google Scholar
[24] Pan, X. B., Condensation of least-energy solutions of a semilinear Neumann problem. J. Partial Differential Equations 8(1995), 1–36.Google Scholar
[25] Wei, J., On the construction of single-peaked solutions of a singularly perturbed semilinear Dirichlet problem. J. Differential Equations 129(1996), 315–333.Google Scholar
[26] Wei, J., On the interior spike layer solutions of singularly perturbed semilinear Neumann problem. Tôhoku Math. J. (2) 50(1998), 159–178.Google Scholar
[27] Wei, J., On the interior spike layer solutions for some singular perturbation problems. Proc. Roy. Soc. Edinburgh, Sect. A 128(1998), 849–874.Google Scholar
[28] Wei, J., On the boundary spike layer solutions of singularly perturbed semilinear Neumann problem. J. Differential Equations 134(1997), 104–133.Google Scholar
[29] Wei, J., On the construction of single interior peak solutions for a singularly perturbed Neumann problem. submitted.Google Scholar
[30] Wei, J. and Winter, M., Stationary solutions for the Cahn-Hilliard equation . Ann. Inst. H. Poincaré Anal.Non Linéaire 15(1998), 459–492.Google Scholar
[31] Wei, J. and Winter, M., On the Cahn-Hilliard equations II: interior spike Layer solutions. J. Differential Equations 148(1998), 231–267.Google Scholar
[32] Wei, J. and Winter, M.,Multiple boundary spike solutions for a wide class of singular perturbation problems. J. LondonMath. Soc., to appear.Google Scholar