Hostname: page-component-6bf8c574d5-86b6f Total loading time: 0 Render date: 2025-03-07T02:47:38.821Z Has data issue: false hasContentIssue false
  • English
  • Français

On several dynamical properties of shifts acting on directed trees

Part of: Normed linear spaces and Banach spaces; Banach lattices General theory of linear operators Graph theory Special classes of linear operators

Published online by Cambridge University Press:  09 January 2025

Evgeny Abakumov  and
Arafat Abbar Open the ORCID record for Arafat Abbar [Opens in a new window]
Evgeny Abakumov
Affiliation:
Univ Gustave Eiffel, Univ Paris Est Creteil, CNRS, LAMA UMR8050 F-77447 Marne-la-Vallée, France e-mail: evgueni.abakoumov@univ-eiffel.fr
Arafat Abbar*
Affiliation:
Univ Gustave Eiffel, Univ Paris Est Creteil, CNRS, LAMA UMR8050 F-77447 Marne-la-Vallée, France e-mail: evgueni.abakoumov@univ-eiffel.fr
*
e-mail: abbar.arafat@gmail.com
Get access Rights & Permissions [Opens in a new window]

Abstract

This article explores the notions of $\mathcal {F}$-transitivity and topological $\mathcal {F}$-recurrence for backward shift operators on weighted $\ell ^p$-spaces and $c_0$-spaces on directed trees, where 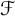$\mathcal {F}$ represents a Furstenberg family of subsets of $\mathbb {N}_0$. In particular, we establish the equivalence between recurrence and hypercyclicity of these operators on unrooted directed trees. For rooted directed trees, a backward shift operator is hypercyclic if and only if it possesses an orbit of a bounded subset that is weakly dense.

Keywords

Backward shiftdirected treehypercyclicityorbital limit pointsrecurrence

MSC classification

Primary: 47A16: Cyclic vectors, hypercyclic and chaotic operators
Secondary: 05C05: Trees 47B37: Operators on special spaces (weighted shifts, operators on sequence spaces, etc.) 46B45: Banach sequence spaces
Type
Article
Information
Canadian Journal of Mathematics , First View , pp. 1 - 30
Check for updates
Copyright
© The Author(s), 2025. Published by Cambridge University Press on behalf of Canadian Mathematical Society

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abakumov, E. and Abbar, A., Orbits of the backward shifts with limit points . J. Math. Anal. Appl. 537(2024), no. 2, 128293.CrossRefGoogle Scholar
Abbar, A. and Kuznetsova, Y., $\varGamma$ -supercyclicity of families of translates in weighted ${L}^p$ -spaces on locally compact groups . J. Math. Anal. Appl. 495(2021), no. 1, 124709.CrossRefGoogle Scholar
Abbar, A., $\varGamma$ -supercyclicity for bilateral shift operators and translation semigroups . Bull. L.N. Gumilyov ENU. Math. Comput. Sci. Mech. Ser. 129(2019), no. 4, 73–79.Google Scholar
Amouch, M., Bachir, A., Benchiheb, O., and Mecheri, S., Weakly recurrent operators . Mediterr. J. Math. 20(2023), no. 3, 169.CrossRefGoogle Scholar
Amouch, M. and Benchiheb, O., On a class of super-recurrent operators . Filomat 36(2022), no. 11, 3701–3708.CrossRefGoogle Scholar
Ángeles-Romero, A. and Martínez-Avendaño, R. A., The forward and backward shift on the Hardy space of a tree , Anal. Math. Phys. 14(2024), no. 70, 37pp.CrossRefGoogle Scholar
Baranov, A., Lishanskii, A., and Papathanasiou, D., Hypercyclic shifts on lattice graphs. Preprint, 2024. arXiv:2312.13934v2.Google Scholar
Bayart, F. and Matheron, E., Dynamics of linear operators . Camb. Tracts Math. 179, Cambridge University Pres, Cambridge (2009).Google Scholar
Bès, J., Menet, Q., Peris, A., and Puig, Y., Strong transitivity properties for operators . J. Diff. Equ. 266(2019), no 2–3, 1313–1337.CrossRefGoogle Scholar
Bermúdez, T., Bonilla, A., and Peris, A., On hypercyclicity and supercyclicity criteria . Bull. Aust. Math. Soc. 70(2004), no. 1, 45–54.CrossRefGoogle Scholar
Bonilla, A., Cardeccia, R., Grosse-Erdmann, K. G., and Muro, S., Zero-one law of orbital limit points for weighted shifts. Preprint, 2024. arXiv:2007.01641v2.Google Scholar
Bonilla, A., Grosse-Erdmann, K. G., López-Martínez, A., and Peris, A., Frequently recurrent operators . J. Funct. Anal. 283(2022), no. 12, 109713.CrossRefGoogle Scholar
Bourbaki, N., General topology: Chapters 1–4, Springer-Verlag, Berlin, Heidelberg, 1995.CrossRefGoogle Scholar
Charpentier, S., Ernst, R., and Menet, Q., Γ-supercyclicity . J. Funct. Anal. 270(2016), no. 12, 4443–4465.CrossRefGoogle Scholar
Costakis, G., Manoussos, A., and Parissis, I., Recurrent linear operators . Complex Anal. Oper. Theory 8(2014), no. 8, 1601–1643.CrossRefGoogle Scholar
Costakis, G. and Parissis, I., Szemerédi’s theorem, frequent hypercyclicity and multiple recurrence . Math. Scand. 110(2012), no. 2, 251–272.CrossRefGoogle Scholar
Chan, K. and Sanders, R., A weakly hypercyclic operator that is not norm hypercyclic . J. Operator Theory 52(2004), no. 1, 39–59.Google Scholar
Chan, K. and Seceleanu, I., Hypercyclicity of shifts as a zero-one law of orbital limit points . J. Operator Theory 67(2012), no. 1, 257–277.Google Scholar
Grivaux, S. and López-Martínez, A., Recurrence properties for linear dynamical systems: an approach via invariant measures . J. Math. Pures Appl. 169(2023), 155–188.CrossRefGoogle Scholar
Grivaux, S., López-Martínez, A., and Peris, A., Questions in linear recurrence: From the $T\oplus T$ -problem to lineability. Preprint, 2023. arXiv:2212.03652v2.CrossRefGoogle Scholar
Grosse-Erdmann, K. G., Hypercyclic and chaotic weighted shifts . Stud. Math. 139(2000), no. 1, 47–68.CrossRefGoogle Scholar
Grosse-Erdmann, K. G. and Papathanasiou, D., Dynamics of weighted shifts on directed trees . Indiana Univ. Math. J. 72(2023), 263–299.CrossRefGoogle Scholar
Grosse-Erdmann, K. G. and Papathanasiou, D., Chaotic weighted shifts on directed trees. Preprint, 2024. arXiv:2303.03980v2.Google Scholar
Grosse-Erdmann, K. G. and Peris, A., Linear Chaos, Springer, London, 2011.CrossRefGoogle Scholar
He, S., Huang, Y., and Yin, Z., $J$ -class weighted backward shifts . Internat. J. Bifur. Chaos 28(2018), no. 06, 1850076.CrossRefGoogle Scholar
Jblónski, Z. J., Jung, I. B., and Stochel, J., Weighted shifts on directed trees . Mem. Am. Math. Soc. 216(2012), no. 1017, viii+107pp.Google Scholar
Martínez-Avendaño, R. A., Hypercyclicity of shifts on weighted Lp spaces of directed trees . J. Math. Anal. Appl. 446(2017), no. 1, 823–842.CrossRefGoogle Scholar
Martínez-Avendaño, R. A. and Rivera-Guasco, E., The forward and backward shift on the Lipschitz space of a tree . Integr. Equ. Oper. Theory 92(2020), no. 1, 1–34.CrossRefGoogle Scholar
Salas, H. N., Hypercyclic weighted shifts . Trans. Amer. Math. Soc. 347(1995), no. 3, 993–1004.CrossRefGoogle Scholar