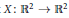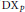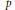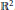Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Chamberland, Marc
2003.
Characterizing Two-Dimensional Maps Whose Jacobians Have Constant Eigenvalues.
Canadian Mathematical Bulletin,
Vol. 46,
Issue. 3,
p.
323.
Fernandes, Alexandre
Gutierrez, Carlos
and
Rabanal, Roland
2004.
Global asymptotic stability for differentiable vector fields of R2.
Journal of Differential Equations,
Vol. 206,
Issue. 2,
p.
470.
Rabanal, Roland
2005.
An eigenvalue condition for the injectivity and asymptotic stability at infinity.
Qualitative Theory of Dynamical Systems,
Vol. 6,
Issue. 2,
p.
233.
Gutierrez, Carlos
Pires, Benito
and
Rabanal, Roland
2006.
Asymptotic stability at infinity for differentiable vector fields of the plane.
Journal of Differential Equations,
Vol. 231,
Issue. 1,
p.
165.
Gutierrez, C.
Jarque, X.
Llibre, J.
and
Teixeira, M. A.
2007.
Global Injectivity ofC1Maps of the Real Plane, Inseparable Leaves and the Palais–Smale Condition.
Canadian Mathematical Bulletin,
Vol. 50,
Issue. 3,
p.
377.
Alarcón, Begoña
Gutierrez, Carlos
and
Martínez-Alfaro, José
2008.
Planar maps whose second iterate has a unique fixed point.
Journal of Difference Equations and Applications,
Vol. 14,
Issue. 4,
p.
421.
Gutierrez, Carlos
and
Maquera, Carlos
2009.
Foliations and polynomial diffeomorphisms of $${\mathbb{R}^{3}}$$.
Mathematische Zeitschrift,
Vol. 262,
Issue. 3,
p.
613.
Pires, Benito
and
Teixeira, Marco Antonio
2012.
On global linearization of planar involutions.
Bulletin of the Brazilian Mathematical Society, New Series,
Vol. 43,
Issue. 4,
p.
637.
Gutierrez, Carlos
Martínez-Alfaro, Jose
and
Venato-Santos, Jean
2012.
Plane foliations with a saddle singularity.
Topology and its Applications,
Vol. 159,
Issue. 2,
p.
484.
Maquera, Carlos
and
Venato-Santos, Jean
2013.
Foliations and global injectivity in ℝ n.
Bulletin of the Brazilian Mathematical Society, New Series,
Vol. 44,
Issue. 2,
p.
273.
FERNANDES, ALEXANDRE
MAQUERA, CARLOS
and
VENATO–SANTOS, JEAN
2014.
Jacobian Conjecture and semi-algebraic maps.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 157,
Issue. 2,
p.
221.
BRAUN, FRANCISCO
and
LLIBRE, JAUME
2015.
A new qualitative proof of a result on the real jacobian conjecture.
Anais da Academia Brasileira de Ciências,
Vol. 87,
Issue. 3,
p.
1519.
Braun, Francisco
Giné, Jaume
and
Llibre, Jaume
2016.
A sufficient condition in order that the real Jacobian conjecture in R2 holds.
Journal of Differential Equations,
Vol. 260,
Issue. 6,
p.
5250.
Mello, Luis Fernando
and
Xavier, Frederico
2020.
Dynamics, points and places at infinity, and the inversion of polynomial self-maps of R2.
Expositiones Mathematicae,
Vol. 38,
Issue. 3,
p.
365.
Llibre, Jaume
and
Valls, Claudia
2021.
A sufficient condition for the real Jacobian conjecture in R2.
Nonlinear Analysis: Real World Applications,
Vol. 60,
Issue. ,
p.
103298.
Giné, Jaume
and
Llibre, Jaume
2021.
A new sufficient condition in order that the real Jacobian conjecture in R2 holds.
Journal of Differential Equations,
Vol. 281,
Issue. ,
p.
333.
Alexandrov, Victor
2021.
Around Efimov’s differential test for homeomorphism.
Beiträge zur Algebra und Geometrie / Contributions to Algebra and Geometry,
Vol. 62,
Issue. 1,
p.
7.
Braun, Francisco
and
Valls, Claudia
2021.
A weight-homogenous condition to the real Jacobian conjecture in .
Proceedings of the Edinburgh Mathematical Society,
Vol. 64,
Issue. 4,
p.
1028.
Valls, Claudia
2024.
A Generalization of the Poincaré Compactification and the Real Jacobian Conjecture.
Journal of Dynamics and Differential Equations,
Vol. 36,
Issue. 1,
p.
619.
Tian, Yuzhou
and
Zhao, Yulin
2024.
On Necessary and Sufficient Conditions for the Real Jacobian Conjecture.
Qualitative Theory of Dynamical Systems,
Vol. 23,
Issue. 1,