Article contents
On the Pointwise Bishop–Phelps–Bollobás Property for Operators
Published online by Cambridge University Press: 17 October 2018
Abstract
We study approximation of operators between Banach spaces 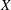 $X$ and
$X$ and 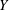 $Y$ that nearly attain their norms in a given point by operators that attain their norms at the same point. When such approximations exist, we say that the pair
$Y$ that nearly attain their norms in a given point by operators that attain their norms at the same point. When such approximations exist, we say that the pair 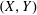 $(X,Y)$ has the pointwise Bishop–Phelps–Bollobás property (pointwise BPB property for short). In this paper we mostly concentrate on those
$(X,Y)$ has the pointwise Bishop–Phelps–Bollobás property (pointwise BPB property for short). In this paper we mostly concentrate on those 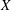 $X$, called universal pointwise BPB domain spaces, such that
$X$, called universal pointwise BPB domain spaces, such that 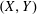 $(X,Y)$ possesses pointwise BPB property for every
$(X,Y)$ possesses pointwise BPB property for every 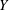 $Y$, and on those
$Y$, and on those 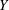 $Y$, called universal pointwise BPB range spaces, such that
$Y$, called universal pointwise BPB range spaces, such that 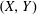 $(X,Y)$ enjoys pointwise BPB property for every uniformly smooth
$(X,Y)$ enjoys pointwise BPB property for every uniformly smooth 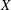 $X$. We show that every universal pointwise BPB domain space is uniformly convex and that
$X$. We show that every universal pointwise BPB domain space is uniformly convex and that 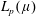 $L_{p}(\unicode[STIX]{x1D707})$ spaces fail to have this property when
$L_{p}(\unicode[STIX]{x1D707})$ spaces fail to have this property when 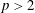 $p>2$. No universal pointwise BPB range space can be simultaneously uniformly convex and uniformly smooth unless its dimension is one. We also discuss a version of the pointwise BPB property for compact operators.
$p>2$. No universal pointwise BPB range space can be simultaneously uniformly convex and uniformly smooth unless its dimension is one. We also discuss a version of the pointwise BPB property for compact operators.
MSC classification
- Type
- Article
- Information
- Copyright
- © Canadian Mathematical Society 2018
Footnotes
The corresponding author is H. J. Lee. The research of the first author was supported by the Centrum pokročilých aplikovaných přírodních věd (Center for Advanced Applied Science) project OPVVV CAAS CZ.02.1.01/0.0/0.0/16_019/0000778, by Pohang Mathematics Institute (PMI), POSTECH, Korea, and by the Basic Science Research Program through the National Research Foundation of Korea (NRF), funded by the Ministry of Education, Science, and Technology (NRF-2015R1D1A1A09059788). The research of the second author was done with the support of the Ukrainian Ministry of Science and Education Research Program 0118U002036, and was partially supported by Spanish MINECO/FEDER projects MTM2015-65020-P and MTM2017-83262-C2-2-P. The third author was partially supported by the Basic Science Research Program through the National Research Foundation of Korea(NRF), funded by the Ministry of Education, Science and Technology (NRF-2017R1C1B1002928). The fourth author was partially supported by the Basic Science Research Program through the National Research Foundation of Korea (NRF), funded by the Ministry of Education, Science and Technology (NRF-2016R1D1A1B03934771). The fifth author was partially supported by Spanish MINECO/FEDER grant MTM2015-65020-P.
References
- 6
- Cited by


