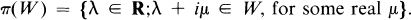Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-27T10:15:20.875Z Has data issue: false hasContentIssue false
  • English
  • Français

On the Spectra of Unbounded Subnormal Operators

Published online by Cambridge University Press:  20 November 2018

G. McDonald  and
C. Sundberg
G. McDonald
Affiliation:
Loyola University, Chicago, Illinois
C. Sundberg
Affiliation:
University of Tennessee, Knoxville, Tennessee
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Putnam showed in [5] that the spectrum of the real part of a bounded subnormal operator on a Hilbert space is precisely the projection of the spectrum of the operator onto the real line. (In fact he proved this more generally for bounded hyponormal operators.) We will show that this result can be extended to the class of unbounded subnormal operators with bounded real parts.

Before proceeding we establish some notation. If T is a (not necessarily bounded) operator on a Hilbert space, then D(T) will denote its domain, and σ(T) its spectrum. For K a subspace of D(T), T|K will denote the restriction of T to K. Norms of bounded operators and elements in Hilbert spaces will be indicated by ‖ ‖. All Hilbert space inner products will be written 〈,〉. If W is a set in C, the closure of W will be written clos W, the topological boundary will be written bdy W, and the projection of W onto the real line will be written π(W),

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 38 , Issue 5 , 01 October 1986 , pp. 1135 - 1148
Copyright
Copyright © Canadian Mathematical Society 1986

References

1. Conway, J. B., Subnormal operators (Pitman Publishing, Boston, 1981).Google Scholar
2. Douglas, R. G., Banach algebra techniques in operator theory (Academic Press, N.Y., 1972).Google Scholar
3. Halmos, P. R., A Hilbert space problem book (D. VanNostrand, Princeton, 1967).Google Scholar
4. McDonald, G. and Sundberg, C., Toeplitz operators on the disc, Indiana University Math. J. 25 (1979), 595–611.Google Scholar
5. Putnam, C. R., On the spectra of semi-normal operators, Transactions A.M.S. 119 (1965), 509–523.Google Scholar
6. Rudin, W., Functional analysis (McGraw-Hill, New York, 1973).Google Scholar