Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-26T16:25:12.117Z Has data issue: false hasContentIssue false
  • English
  • Français

On the Weak Order of Coxeter Groups

Part of: Lie algebras and Lie superalgebras Special aspects of infinite or finite groups Lattices

Published online by Cambridge University Press:  10 January 2019

Matthew Dyer
Matthew Dyer*
Affiliation:
Department of Mathematics, 255 Hurley Building, University of Notre Dame, Notre Dame, Indiana 46556, USA Email: dyer.1@nd.edu
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

This paper provides some evidence for conjectural relations between extensions of (right) weak order on Coxeter groups, closure operators on root systems, and Bruhat order. The conjecture focused upon here refines an earlier question as to whether the set of initial sections of reflection orders, ordered by inclusion, forms a complete lattice. Meet and join in weak order are described in terms of a suitable closure operator. 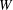Galois connections are defined from the power set of $W$ to itself, under which maximal subgroups of certain groupoids correspond to certain complete meet subsemilattices of weak order. An analogue of weak order for standard parabolic subsets of any rank of the root system is defined, reducing to the usual weak order in rank zero, and having some analogous properties in rank one (and conjecturally in general).

Keywords

Coxeter grouproot systemweak orderlattice

MSC classification

Primary: 20F55: Reflection and Coxeter groups
Secondary: 06B23: Complete lattices, completions 17B22: Root systems
Type
Article
Information
Canadian Journal of Mathematics , Volume 71 , Issue 2 , April 2019 , pp. 299 - 336
Copyright
© Canadian Mathematical Society 2018 

References

Björner, Anders and Brenti, Francesco, Combinatorics of Coxeter groups. Graduate Texts in Mathematics, 231. Springer, New York, 2005.Google Scholar
Björner, Anders, Edelman, Paul H., and Ziegler, Günter M., Hyperplane arrangements with a lattice of regions . Discrete Comput. Geom. 5(1990), no. 3, 263–288. https://doi.org/10.1007/BF02187790.Google Scholar
Björner, Anders, Las Vergnas, Michel, Sturmfels, Bernd, White, Neil, and Ziegler, Günter M., Oriented matroids. Encyclopedia of Mathematics and its Applications, 46. Cambridge University Press, Cambridge, 1999. https://doi.org/10.1017/CBO9780511586507.Google Scholar
Bourbaki, N., Éléments de mathématique. Fasc. XXXIV. Groupes et algèbres de Lie. Chapitre IV: Groupes de Coxeter et systèmes de Tits. Chapitre V: Groupes engendrés par des réflexions. Chapitre VI: systèmes de racines. Actualités Scientifiques et Industrielles, 1337. Hermann, Paris, 1968.Google Scholar
Brink, Brigitte, On centralizers of reflections in Coxeter groups . Bull. London Math. Soc. 28(1996), no. 5, 465–470. https://doi.org/10.1112/blms/28.5.465.Google Scholar
Brink, Brigitte and Howlett, Robert B., Normalizers of parabolic subgroups in Coxeter groups . Invent. Math. 136(1999), no. 2, 323–351. https://doi.org/10.1007/s002220050312.Google Scholar
Büchi, J. Richard and Fenton, William E., Large convex sets in oriented matroids . J. Combin. Theory Ser. B 45(1988), no. 3, 293–304. https://doi.org/10.1016/0095-8956(88)90074-3.Google Scholar
Cuntz, M. and Heckenberger, I., Weyl groupoids with at most three objects . J. Pure Appl. Algebra 213(2009), no. 6, 1112–1128. https://doi.org/10.1016/j.jpaa.2008.11.009.Google Scholar
Davey, B. A. and Priestley, H. A., Introduction to lattices and order. Second edition. Cambridge University Press, New York, 2002. https://doi.org/10.1017/CBO9780511809088.Google Scholar
Ðoković, D. Ž., Check, P., and Hée, J.-Y., On closed subsets of root systems . Canad. Math. Bull. 37(1994), no. 3, 338–345. https://doi.org/10.4153/CMB-1994-050-4.Google Scholar
Dyer, M. J., Reflection subgroups of Coxeter systems . J. Algebra 135(1990), no. 1, 57–73. https://doi.org/10.1016/0021-8693(90)90149-I.Google Scholar
Dyer, M. J., On the “Bruhat graph” of a Coxeter system . Compositio Math. 78(1991), no. 2, 185–191.Google Scholar
Dyer, M. J., Hecke algebras and shellings of Bruhat intervals. II. Twisted Bruhat orders . In: Kazhdan-Lusztig theory and related topics. Contemp. Math., 139. American Mathematical Society, Providence, RI, 1992, pp. 141–165. https://doi.org/10.1090/conm/139/1197833.Google Scholar
Dyer, M. J., Hecke algebras and shellings of Bruhat intervals . Compositio Math. 89(1993), no. 1, 91–115.Google Scholar
Dyer, M. J., Bruhat intervals, polyhedral cones and Kazhdan-Lusztig-Stanley polynomials . Math. Z. 215(1994), no. 2, 223–236. 1994. https://doi.org/10.1007/BF02571712.Google Scholar
Dyer, M. J., Quotients of twisted Bruhat orders . J. Algebra 163(1994), no. 3, 861–879. https://doi.org/10.1006/jabr.1994.1049.Google Scholar
Dyer, M. J., On rigidity of abstract root systems of Coxeter groups. arxiv:1011.2270[math.GR] 2010.Google Scholar
Dyer, Matthew and Bonnafé, Cedric, Semidirect product decompositions of Coxeter groups . Comm. Algebra 38(2010), no. 4, 1549–1574. https://doi.org/10.1080/00927870902980354.Google Scholar
Edelman, Paul H., Meet-distributive lattices and the anti-exchange closure . Algebra Universalis 10(1980), no. 3, 290–299. https://doi.org/10.1007/BF02482912.Google Scholar
Edgar, Tom, Sets of reflections defining twisted Bruhat orders . J. Algebraic Combin. 26(2007), no. 3, 357–362. https://doi.org/10.1007/s10801-007-0060-9.Google Scholar
Heckenberger, I. and Welker, W., Geometric combinatorics of Weyl groupoids. arxiv:1003.3231[math.QA], 2010.Google Scholar
Heckenberger, István and Yamane, Hiroyuki, A generalization of Coxeter groups, root systems, and Matsumoto’s theorem . Math. Z. 259(2008), no. 2, 255–276. https://doi.org/10.1007/s00209-007-0223-3.Google Scholar
Humphreys, James E., Reflection groups and Coxeter groups. Cambridge Studies in Advanced Mathematics, 29. Cambridge University Press, Cambridge, 1990. https://doi.org/10.1017/CBO9780511623646.Google Scholar
MacLane, Saunders, Categories for the working mathematician. Graduate Texts in Mathematics, 5. Springer-Verlag, New York, 1998.Google Scholar
Malyšev, F. M., Decomposition of root systems . Mat. Zametki 27(1980), no. 6, 869–876.Google Scholar
Pilkington, Annette, Convex geometries on root systems . Comm. Algebra 34(2006), no. 9, 3183–3202. https://doi.org/10.1080/00927870600778340.Google Scholar
Wang, Weijia, Closure operator and lattice property of root systems. Ph.D. thesis, University of Notre Dame, 2017.Google Scholar