Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-15T18:33:34.572Z Has data issue: false hasContentIssue false
  • English
  • Français

Operators of the form PAQ-QAP

Published online by Cambridge University Press:  20 November 2018

Arlen Brown  and
Carl Pearcy
Arlen Brown
Affiliation:
University of Michigan, Ann Arbor, Michigan
Carl Pearcy
Affiliation:
University of Michigan, Ann Arbor, Michigan
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this note the Hilbert spaces under consideration are complex, and the operators referred to are bounded, linear operators. If 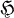is a Hilbert space, then the algebra of all operators on 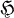is denoted by .

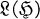It is known (1) that if 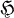is any Hilbert space, then the class of commutators on 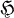, i.e., the class of all operators that can be written in the form PQ — QP for some 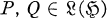, can be exactly described. A similar problem is that of characterizing all operators on 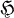that can be written in the form 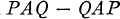for some .

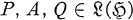Type
Research Article
Information
Canadian Journal of Mathematics , Volume 20 , 1968 , pp. 1353 - 1361
Copyright
Copyright © Canadian Mathematical Society 1968

Footnotes

This research was supported in part by the National Science Foundation. At the time the paper was written, the second author was an Alfred P. Sloan Research Fellow.

References

1. Brown, A. and Pearcy, C., Structure of commutators of operators, Ann. of Math. (2) 82 (1965), 112–127.Google Scholar
2. Brown, A., Halmos, P. R., and Pearcy, C., Commutators of operators on Hilbert space, Can. J. Math. 17 (1965), 695–708.Google Scholar
3. Lumer, G. and Rosenblum, M., Linear operator equations, Proc. Amer. Math. Soc. 10 (1959), 32–41.Google Scholar
4. Taussky, O., Generalized commutators of matrices and permutations of factors in a product of three matrices (Studies in Mathematics and Mechanics presented to Richard von Mises, Academic Press, New York, 1954).Google Scholar