No CrossRef data available.
Published online by Cambridge University Press: 20 November 2018
We obtain a number of new conditions for oscillation of the first order neutral delay equation with nonconstant coefficients of the form
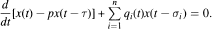
Comparison results are also given as well as conditions for the existence of nonoscillatory solutions.