Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Louboutin, S.
Mollin, R. A.
and
Williams, H. C.
1992.
Class Numbers of Real Quadratic Fields, Continued Fractions, Reduced Ideals, Prime-Producing Quadratic Polynomials and Quadratic Residue Covers.
Canadian Journal of Mathematics,
Vol. 44,
Issue. 4,
p.
824.
Louboutin, Stéphane
1993.
Minorations d’unités fondamentales—applications.
Nagoya Mathematical Journal,
Vol. 130,
Issue. ,
p.
1.
Zhang, Ming Yao
1995.
On Yokoi’s conjecture.
Mathematics of Computation,
Vol. 64,
Issue. 212,
p.
1675.
2006.
Die Welt der Primzahlen.
p.
135.
Ribenboim, Paulo
2011.
Die Welt der Primzahlen.
p.
137.
Biró, András
and
Granville, Andrew
2012.
Zeta functions for ideal classes in real quadratic fields, ats=0.
Journal of Number Theory,
Vol. 132,
Issue. 8,
p.
1807.
Hoque, Azizul
and
Kotyada, Srinivas
2021.
Class number one problem for the real quadratic fields $${{\mathbb {Q}({\sqrt{m^2+2r}})}}$$.
Archiv der Mathematik,
Vol. 116,
Issue. 1,
p.
33.
Mahapatra, Nimish Kumar
Pandey, Prem Prakash
and
Ram, Mahesh Kumar
2023.
Fields of small class number in the family $$\mathbb {Q}(\sqrt{9m^2+4m})$$.
The Ramanujan Journal,
Vol. 61,
Issue. 3,
p.
779.
Gica, Alexandru
2024.
Class numbers, Ono invariants and some interesting primes.
Indagationes Mathematicae,
Vol. 35,
Issue. 6,
p.
1249.
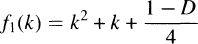 with discriminant Don consecutive integers (See [1], [7]). M. D. Hendy extended Frobenius-Rabinowitsch's result to a necessary and sufficient condition for the class-number of a complex quadratic field with discriminant D to be two in terms of the primality of the values taken by the quadratic polynomials
with discriminant Don consecutive integers (See [1], [7]). M. D. Hendy extended Frobenius-Rabinowitsch's result to a necessary and sufficient condition for the class-number of a complex quadratic field with discriminant D to be two in terms of the primality of the values taken by the quadratic polynomials 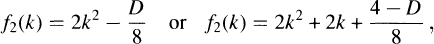 and
and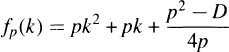 with discriminant D (see [2], [7]).
with discriminant D (see [2], [7]).