Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Gbur, Mary E.
1976.
On the lower Markov spectrum.
Monatshefte f�r Mathematik,
Vol. 81,
Issue. 2,
p.
95.
Gbur, Mary E.
1977.
Accumulation points of the Lagrange and Markov spectra.
Monatshefte für Mathematik,
Vol. 84,
Issue. 2,
p.
91.
Malyshev, A. V.
1981.
Markov and Lagrange spectra (survey of the literature).
Journal of Soviet Mathematics,
Vol. 16,
Issue. 1,
p.
767.
Ramharter, Gerhard
1982.
Über asymmetrische diophantische approximationen.
Journal of Number Theory,
Vol. 14,
Issue. 2,
p.
269.
Dietz, Bernhard
1983.
On the gaps of the Markoff spectrum.
Monatshefte f�r Mathematik,
Vol. 96,
Issue. 4,
p.
265.
Cusick, T. W.
1987.
Endpoints of gaps in the Markoff spectrum.
Monatshefte für Mathematik,
Vol. 103,
Issue. 2,
p.
85.
1987.
Geometry of Numbers.
Vol. 37,
Issue. ,
p.
632.
Crisp, David
and
Moran, William
2017.
Number Theory with an Emphasis on the Markoff Spectrum.
p.
83.

 , where the
, where the 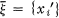 , where
, where 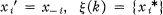 where
where 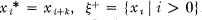 . We let
. We let 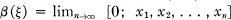 = [0;
= [0;