Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
1980.
Group Representations.
Vol. 180,
Issue. ,
p.
849.
1987.
Representations of Finite Groups.
p.
407.
1992.
Group Representations Volume 1 Part B: Introduction to Group Representations and Characters.
Vol. 175,
Issue. ,
p.
1231.
1996.
Vol. 183,
Issue. ,
p.
889.



 :
:
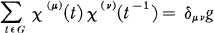

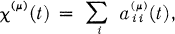
 is 1 or 0 according as t and s are conjugate in
is 1 or 0 according as t and s are conjugate in 