Hostname: page-component-78c5997874-g7gxr Total loading time: 0 Render date: 2024-11-15T22:35:09.239Z Has data issue: false hasContentIssue false
  • English
  • Français

The Representation of a Graph by Set Intersections

Published online by Cambridge University Press:  20 November 2018

Paul Erdös ,
A. W. Goodman  and
Louis Pósa
Paul Erdös
Affiliation:
University of Alberta, Edmonton, The University of South Florida and Michael Fazekas High School, Budapest
A. W. Goodman
Affiliation:
University of Alberta, Edmonton, The University of South Florida and Michael Fazekas High School, Budapest
Louis Pósa
Affiliation:
University of Alberta, Edmonton, The University of South Florida and Michael Fazekas High School, Budapest
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Geometrically, a graph is a collection of points (or vertices) together with a set of edges (or curves) each of which joins two distinct vertices of the graph, and no two of which have points in common except possibly end points. Two given vertices of the graph may be joined by no edge or one edge, but may not be joined by more than one edge. From an abstract point of view, a graph G is a collection of elements ﹛x1, x2, …﹜ called points or vertices, together with a second collection of certain pairs (xα, Xβ) of distinct points of G. It is helpful to retain the geometric language, and refer to any pair in 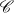as an edge (or a curve) of G that joins the points xα and Xβ.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 18 , 1966 , pp. 106 - 112
Copyright
Copyright © Canadian Mathematical Society 1966

References

1. Čulik, K., Applications of graph theory to mathematical logic and linguistics, Proc. Symp. Graph Theory, Smolenice (1963), 13–20.Google Scholar
2. Szpilrajn-Marczewski, E., Sur deux propriétés des classes d'ensembles, Fund. Math., 33 (1945), 303–307.Google Scholar
3. Turan, P., On the theory of graphs, Colloq. Math., 3 (1954), 19–30.Google Scholar