Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-27T08:10:52.526Z Has data issue: false hasContentIssue false
  • English
  • Français

Some Transformations of Hausdorff Moment Sequences and Harmonic Numbers

Published online by Cambridge University Press:  20 November 2018

Christian Berg  and
Antonio J. Durán
Christian Berg
Affiliation:
Institut for Matematiske Fag., Københavns Universitet, Universitetsparken 5, DK-2100 København ø, Denmark, e-mail: berg@math.ku.dk
Antonio J. Durán
Affiliation:
Departamento de Análisis Matemático, Universidad de Sevilla, Apdo (P. O. BOX) 1160, 41080 Sevilla, Spain, e-mail: duran@us.es
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

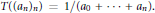We introduce some non-linear transformations from the set of Hausdorff moment sequences into itself; among them is the one defined by the formula: $T\left( {{\left( {{a}_{n}} \right)}_{n}} \right)\,=\,1/\left( {{a}_{0}}\,+\cdots +\,{{a}_{n}} \right).$ We give some examples of Hausdorff moment sequences arising from the transformations and provide the corresponding measures: one of these sequences is the reciprocal of the harmonic numbers 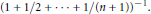${{\left( 1+1/2\,+\cdots +\,1/\left( n+1 \right) \right)}^{-1}}.$

Keywords

Primary: 44A60secondary: 40B05
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 57 , Issue 5 , 01 October 2005 , pp. 941 - 960
Copyright
Copyright © Canadian Mathematical Society 2005

References

[B] Berg, C., Quelques remarques sur le cône de Stieltjes. In: Seminar on Potential Theory, Lecture Notes in Math. 814, Springer-Verlag, Berlin, 1980.Google Scholar
[BCR] Berg, C., Christensen, J. P. R., and Ressel, P., Harmonic analysis on semigroups. In: Theory of Positive Definite and Related Functions, Graduate Texts in Mathematics 100, Springer-Verlag, New York, 1984.Google Scholar
[BD] Berg, C. and Durán, A. J., A transformation from Hausdorff to Stieltjes moment sequences. Ark. Mat. 42(2004), 239–257.Google Scholar
[BF] Berg, C. and Forst, G., Potential theory on locally compact abelian groups. Ergebnisse der Mathematik und ihrer Grenzgebiete 87. Springer-Verlag, New York, 1975.Google Scholar
[Bt] Bertoin, J., Lévy Processes. Cambridge Tracts in Mathematics 121, Cambridge University Press, Cambridge, 1996.Google Scholar
[D] Donoghue, W. F., Jr., MonotoneMatrix Functions and Analytic Continuation. Die Grundlehren der mathematischenWissenschaften 207, Springer-Verlag, New York, 1974.Google Scholar
[GR] Gradshteyn, I. S. and Ryzhik, I. M., Table of Integrals, Series And Products. Academic Press, New York, 1980.Google Scholar
[GKP] Graham, R. L., Knuth, D. E., and Patashnik, O., Concrete Mathematics: A Foundation For Computer Science. Second ed. Addison, Wesley, Reading, MA: 1994.Google Scholar
[H] Hausdorff, F.,Momentprobleme für ein endliches Intervall. Math. Z. 16(1923) 220–248.Google Scholar
[I] It ô, M., Sur les cônes convexes de Riesz et les noyaux complètement sous-harmoniques. Nagoya Math. J. 55(1974), 111–144.Google Scholar
[R] Reuter, G. E. H., Über eine Volterrasche Integralgleichung mit totalmonotonem Kern. Arch. Math. 7(1956), 59–66.Google Scholar