Article contents
Topologizing Different Classes of Real Functions
Published online by Cambridge University Press: 20 November 2018
Abstract
The purpose of this paper is to examine which classes  of functions from
of functions from  can be topologized in a sense that there exist topologies τ1 and τ2 on
can be topologized in a sense that there exist topologies τ1 and τ2 on 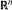 and
and 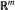 respectively, such that
respectively, such that  is equal to the class C(τ1 , τ2) of all continuous functions
is equal to the class C(τ1 , τ2) of all continuous functions 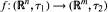 . We will show that the Generalized Continuum Hypothesis GCH implies the positive answer for this question for a large number of classes of functions
. We will show that the Generalized Continuum Hypothesis GCH implies the positive answer for this question for a large number of classes of functions  for which the sets {x : f(x) = g(x)} are small in some sense for all f, g ∈
for which the sets {x : f(x) = g(x)} are small in some sense for all f, g ∈  f ≠ g. The topologies will be Hausdorff and connected. It will be also shown that in some model of set theory ZFC with GCH these topologies could be completely regular and Baire. One of the corollaries of this theorem is that GCH implies the existence of a connected Hausdorff topology T on
f ≠ g. The topologies will be Hausdorff and connected. It will be also shown that in some model of set theory ZFC with GCH these topologies could be completely regular and Baire. One of the corollaries of this theorem is that GCH implies the existence of a connected Hausdorff topology T on  such that the class L of all linear functions g(x) = ax + b coincides with
such that the class L of all linear functions g(x) = ax + b coincides with 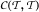 . This gives an affirmative answer to a question of Sam Nadler. The above corollary remains true for the class
. This gives an affirmative answer to a question of Sam Nadler. The above corollary remains true for the class  of all polynomials, the class
of all polynomials, the class  of all analytic functions and the class of all harmonic functions.
of all analytic functions and the class of all harmonic functions.
We will also prove that several other classes of real functions cannot be topologized. This includes the classes of C∞ functions, differentiable functions, Darboux functions and derivatives.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1994
References
- 2
- Cited by


