Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-30T23:21:49.425Z Has data issue: false hasContentIssue false
  • English
  • Français

The Torsion Free Pieri Formula

Published online by Cambridge University Press:  20 November 2018

D. J. Britten  and
F. W. Lemire
D. J. Britten
Affiliation:
Department of Mathematics University of Windsor Windsor, Ontario N9B 3P4
F. W. Lemire
Affiliation:
Department of Mathematics University of Windsor Windsor, Ontario N9B 3P4
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Central to the study of simple infinite dimensional 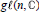$g\ell (n,\,\mathbb{C})$-modules having finite dimensional weight spaces are the torsion free modules. All degree 1 torsion free modules are known. Torsion free modules of arbitrary degree can be constructed by tensoring torsion free modules of degree 1 with finite dimensional simple modules. In this paper, the central characters of such a tensor product module are shown to be given by a Pieri-like formula, complete reducibility is established when these central characters are distinct and an example is presented illustrating the existence of a nonsimple indecomposable submodule when these characters are not distinct.

Keywords

17B10
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 50 , Issue 2 , 01 April 1998 , pp. 266 - 289
Copyright
Copyright © Canadian Mathematical Society 1998

References

[BBL1] Benkart, G.M., Britten, D.J. and Lemire, F.W., Stability in Modules for Classical Lie Algebras—A Constructive Approach. Mem. Amer.Math. Soc. 85(1990).Google Scholar
[BBL2] Benkart, G.M.,Britten, D.J. and Lemire, F.W., Modules with Bounded Weight Multiplicities for Simple Lie Algebras. Math. Z., to appear.Google Scholar
[BFL] Britten, D.J., Futorny, V. and Lemire, F.W., Simple A2 modules with a finite dimensional weight space. Comm. Algebra 23(1995), 467–510.Google Scholar
[BHL] Britten, D.J., Hooper, J. and Lemire, F.W., Simple Cn modules with multiplicities 1 and applications. Canad. J. Phys. 72(1994), 326–335.Google Scholar
[BL1] Britten, D.J. and Lemire, F.W., A classification of simple Lie modules having a 1-dimensional weight space. Trans. Amer.Math. Soc. 299(1987), 683–697.Google Scholar
[BL2] Britten, D.J., On basic cycles of An, Bn, Cn and Dn. Canad. J. Math. 37(1985), 122–140.Google Scholar
[C] Chen, L., Simple torsion free C2-modules having a 1-dimensional weight space. M. Sc. Thesis, University of Windsor, Windsor, Ontario, 1995.Google Scholar
[D] Dixmier, J., Algébres Enveloppantes. Gauthier-Villars, Paris, Brussels, Montréal, 1974.Google Scholar
[DOF] Drozd, Y.A., Ovsienko, S.A. and Futorny, V.M., On Gel’fand-Zetlin Modules. Proc. of the Winter School on Geometry and Physics, Series II 26(1991), 143–147.Google Scholar
[Du] Duflo, M., Sur la classification des idéaux primitifs dans l’alg`ebre enveloppante d’une alg`ebre de Lie semi-simple. Ann. of Math. 105(1977), 107-120.Google Scholar
[F] Fernando, S.L., Lie algebra modules with finite dimensional weight spaces I. Trans. Amer. Math. Soc. 322(1990), 757–781.Google Scholar
[FH] Fulton, W. and Harris, J., Representation Theory—A First Course. Graduate Texts in Math. 129, Springer- Verlag, New York, 1991.Google Scholar
[H] Humphreys, J.E., Introduction to Lie Algebras and Representation Theory. Graduate Texts in Math. 9. Springer-Verlag, New York, 1972.Google Scholar
[K] Kostant, B., On the tensor product of a finite and an infinite dimensional representation. J. Funct. Anal. 20(1975), 257–285.Google Scholar
[L1] Lemire, F.W., Irreducible representations of a simple Lie algebra admitting a one-dimensional weight space. Proc. Amer. Math. Soc. 19(1968), 1161–1164.Google Scholar
[L2] Lemire, F.W., Weight Spaces and Irreducible Representations of Simple Lie Algebras. Proc. Amer.Math. Soc. 22(1969), 192–197.Google Scholar
[L3] Lemire, F.W., One dimensional representations of the cycle subalgebra of a semisimple Lie algebra. Canad. Math. Bull. 13(1970), 463–467.Google Scholar
[Z] Zelobenko, D.P.,Compact Lie Groups and their Representations. Translations of Math. Monograph. 40, Amer. Math. Soc., Providence, Rhode Island, 1973Google Scholar