Hostname: page-component-78c5997874-lj6df Total loading time: 0 Render date: 2024-11-10T17:26:59.738Z Has data issue: false hasContentIssue false
  • English
  • Français

Tropical geometry and Newton–Okounkov cones for Grassmannian of planes from compactifications

Part of: Special varieties Representation theory of rings and algebras

Published online by Cambridge University Press:  12 October 2020

Christopher Manon  and
Jihyeon Jessie Yang
Christopher Manon
Affiliation:
University of Kentucky, Lexington, KY, USA e-mail: Christopher.Manon@uky.edu
Jihyeon Jessie Yang*
Affiliation:
Department of Mathematical and Computational Sciences, University of Toronto Mississauga, Mississauga, ON, Canada
*
e-mail: jessie.yang@utoronto.ca
Get access Rights & Permissions [Opens in a new window]

Abstract

We construct a family of compactifications of the affine cone of the Grassmannian variety of 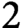$2$ -planes. We show that both the tropical variety of the Plücker ideal and familiar valuations associated to the construction of Newton–Okounkov bodies for the Grassmannian variety can be recovered from these compactifications. In this way, we unite various perspectives for constructing toric degenerations of flag varieties.

Keywords

Compactificationtropical geometryNewton–Okounkov body

MSC classification

Primary: 14M15: Grassmannians, Schubert varieties, flag manifolds 14M25: Toric varieties, Newton polyhedra 14M27: Compactifications; symmetric and spherical varieties 14T05: Tropical geometry
Secondary: 16G20: Representations of quivers and partially ordered sets
Type
Article
Information
Canadian Journal of Mathematics , Volume 74 , Issue 1 , February 2022 , pp. 199 - 231
Check for updates
Copyright
© Canadian Mathematical Society 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

C.M. was supported by the NSF (DMS 1500966) and a Simons Collaboration Grant.

References

Alexeev, V. and Brion, M., Toric degenerations of spherical varieties. Selecta Math. (N.S.) 10(2004), no. 4, 453–478.CrossRefGoogle Scholar
Berkovich, V. G., Spectral theory and analytic geometry over non-Archimedean fields. Mathematical Surveys and Monographs, 33, Amer. Math. Soc., Providence, RI, 1990.Google Scholar
Billera, L. J., Holmes, S. P., and Vogtmann, K., Geometry of the space of phylogenetic trees. Adv. Appl. Math. 27(2001), no. 4, 733–767.CrossRefGoogle Scholar
Bossinger, L., Fang, X., Fourier, G., Hering, M., and Lanini, M., Toric degenerations of $Gr\left(2,n\right)$ and $Gr\left(3,6\right)$ via plabic graphs. Ann. Comb. 22(2018), 491–512.CrossRefGoogle Scholar
Bruns, W. and Herzog, J., Cohen-Macaulay rings . Cambridge Studies in Advanced Mathematics, 39, Cambridge University Press, Cambridge, UK, 1993.Google Scholar
Cueto, M. A., Häbich, M., and Werner, A., Faithful tropicalization of the Grassmannian of planes. Math. Ann. 360(2014), nos. 1–2, 391–437.CrossRefGoogle Scholar
Dolgachev, I., Lectures on invariant theory . London Mathematical Society Lecture Note Series, 296, Cambridge University Press, Cambridge, UK, 2003.CrossRefGoogle Scholar
Eisenbud, D. and Sturmfels, B., Binomial ideals. Duke Math. J. 84(1996), no. 1, 1–45.CrossRefGoogle Scholar
Fang, X., Fourier, G., and Littelmann, P., Essential bases and toric degenerations arising from birational sequences. Adv. Math. 312(2017), no. 25, 107–149.CrossRefGoogle Scholar
Fang, X., Fourier, G., and Littelmann, P., Favourable modules: filtrations, polytopes, Newton-Okounkov bodies and flat degenerations. Transform. Groups 22(2017), 321–352.Google Scholar
Fang, X., Fourier, G., and Littelmann, P., On toric degenerations of flag varieties. In: Krause, H., Littlemann, P., Malle, G., Neeb, K.-H., and Schweigert, C. (eds.), Representation theory: current trends and perspectives, EMS Series of Congress Reports, European Mathematical Society, Zürich, Switzerland, 2017, pp. 187–232.CrossRefGoogle Scholar
Fulton, W. and Harris, J., Representation theory . Graduate Texts in Mathematics, 129, Springer-Verlag, New York, NY, 1991. A first course, Readings in Mathematics.Google Scholar
Gross, M., Hacking, P., Keel, S., and Kontsevich, M., Canonical bases for cluster algebras. J. Amer. Math. Soc. 31(2018), no. 2, 497–608.CrossRefGoogle Scholar
Hilgert, J., Manon, C., and Martens, J., Contraction of hamiltonian $K$ -spaces. Int. Math. Res. Not. 20(2017), no. 1, 6255–6309.Google Scholar
Howard, B., Manon, C., and Millson, J., The toric geometry of triangulated polygons in Euclidean space. Can. J. Math. 63(2011), no. 4, 878–937.CrossRefGoogle Scholar
Howard, B., Millson, J., Snowden, A., and Vakil, R., The equations for the moduli space of $n$ points on the line. Duke Math. J. 146(2009), no. 2, 175–226.CrossRefGoogle Scholar
Kaveh, K., Crystal bases and Newton-Okounkov bodies. Duke Math. J. 164(2015), no. 13, 2461–2506.CrossRefGoogle Scholar
Kaveh, K. and Khovanskii, A. G., Newton-Okounkov bodies, semigroups of integral points, graded algebras and intersection theory. Ann. Math. 176(2012), no. 2, 925–978.CrossRefGoogle Scholar
Kaveh, K. and Manon, C., Khovanskii bases, higher rank valuations, and tropical geometry. SIAM J. Appl. Algebra Geom. 3(2019), no. 2, 292–336.CrossRefGoogle Scholar
Lazarsfeld, R. and Mustaţă, M., Convex bodies associated to linear series . Ann. Sci. Éc Norm. Supér. 42(2009), no. 5, 783–835.CrossRefGoogle Scholar
Lawton, S. and Manon, C., Character varieties of free groups are Gorenstein but not always factorial. J. Algebra 456(2016), 278–293.CrossRefGoogle Scholar
Manon, C., Dissimilarity maps on trees and the representation theory of ${SL}_m\left(\mathbb{C}\right)$ . J. Algebraic Combin. 33(2011), no. 2, 199–213.CrossRefGoogle Scholar
Manon, C., Dissimilarity maps on trees and the representation theory of $G{L}_n\left(\mathbb{C}\right)$ . Electron. J. Comb. 19(2012), no. 3, 12.Google Scholar
Manon, C., Newton-Okounkov polyhedra for character varieties and configuration spaces. Trans. Amer. Math. Soc. 368(2016), no. 8, 5979–6003.CrossRefGoogle Scholar
Manon, C., Toric geometry of free group sl2 character varieties from outer space. Can. J. Math. 70(2018), no. 2, 354–399.CrossRefGoogle Scholar
Maclagan, D. and Sturmfels, B., Introduction to tropical geometry . Graduate Studies in Mathematics, 161, Amer. Math. Soc., Providence, RI, 2015.CrossRefGoogle Scholar
Manon, C. and Zhou, Z., Semigroups of $s{l}_3\left(\mathbb{C}\right)$ tensor product invariants. J. Algebra 400(2014), 94–104.CrossRefGoogle Scholar
Payne, S., Analytification is the limit of all tropicalizations. Math. Res. Lett. 16(2009), no. 3, 543–556.CrossRefGoogle Scholar
Popov, V. L., Contraction of the actions of reductive algebraic groups. Sbornik Math. 2(1987), no. 58, 311–335.CrossRefGoogle Scholar
Pachter, L. and Speyer, D., Reconstructing trees from subtree weights. Appl. Math. Lett. 17(2004), no. 6, 615–621.CrossRefGoogle Scholar
Rietsch, K. and Williams, L., Newton-Okounkov bodies, cluster duality, and mirror symmetry for Grassmannians. Duke Math. J. 168(2019), no. 18, 3437–3527.CrossRefGoogle Scholar
Speyer, D. and Sturmfels, B., The tropical Grassmannian. Adv. Geom. 4(2004), no. 3, 389–411.CrossRefGoogle Scholar
Sturmfels, B., Gröbner bases and convex polytopes. University Lecture Series, 8, Amer. Math. Soc., Providence, RI, 1996.Google Scholar
Timashev, D., Homogeneous spaces and equivariant embeddings. Vol. 8. Encyclopedia of Mathematical Sciences, 138, Invariant Theory and Algebraic Transformation Groups, Springer, Heidelberg, Germany, 2011.CrossRefGoogle Scholar
Watanabe, K., Some remarks concerning Demazure’s construction of normal graded rings. Nagoya Math. J. 83(1981), 203–211.CrossRefGoogle Scholar