Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-26T06:32:12.193Z Has data issue: false hasContentIssue false
  • English
  • Français

When is Every Kernel Functor Idempotent?

Published online by Cambridge University Press:  20 November 2018

Jorge E. Viola-Prioli
Jorge E. Viola-Prioli*
Affiliation:
Universidad de Oriente, Cutnana, Venezuela
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Introduction. All rings occurring are associative and possess a unity, which is preserved under subrings and ring homomorphisms. All modules are unitary right modules. We 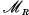denote the category of rights-modules.

In recent years several authors have studied rings R by imposing restrictions on the torsion theories [4] of 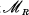. (See for instance [2; 23; 24].) This paper offers another alternative to that trend, namely the study of rings R via their set of kernel functors K﹛R).

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 27 , Issue 3 , 01 June 1975 , pp. 545 - 554
Copyright
Copyright © Canadian Mathematical Society 1975

References

1. Cartan, H. and Eilenberg, S., Homological algebra (Princeton University Press, Princeton, N.J., 1956).Google Scholar
2. Cateforis, V. and Sandomierski, F., The singular submodule splits off, J. Algebra 10 (1968), 149–165.Google Scholar
3. Cozzens, J., Homological properties of the ring of differential polynomials, Bull. Amer. Math. Soc. 76 (1970), 75–79.Google Scholar
4. Dickson, S., A torsion theory for abelian categories, Trans. Amer. Math. Soc. 121 (1966), 223–235.Google Scholar
5. Faith, C., A correspondence theorem for projective modules and the structure of simple noetherian rings, Instituto Nazionale di Alta Matematica, Symposia Mathematica, Vol. VIII (1972), 309–345.Google Scholar
6. Faith, C., Lectures on infective modules and quotient rings, Lecture Notes in Mathematics No. 49 (Springer Verlag, Berlin and New York, 1967).Google Scholar
7. Faith, C., When are proper cyclics infective. Pacific J. Math. 45 (1973), 97–112.Google Scholar
8. Faith, C. and Utumi, Y., On noetherian prime rings, Trans. Am. Math. Soc. 114 (1965), 53–60.Google Scholar
9. Gabriel, P., Des catégories abéliennes, Bull. Soc. Math. France 90 (1962), 323–448.Google Scholar
10. Gardner, B., Rings whose modules form few torsion classes, Bull. Austral. Math. Soc. 4 (1971), 355–359.Google Scholar
11. Goldie, A., Semi-prime rings with maximum condition, Proc. Lond. Math. Soc. 10 (1960), 201–220.Google Scholar
12. Goldman, O., Rings and modules of quotients, J. Algebra 13 (1969), 10–47.Google Scholar
13. Goodearl, Am. Math. Soc. Memoirs No.Google Scholar
14. Jacobson, N., Structure of rings (Amer. Math. Soc, Providence, R.I., 1964).Google Scholar
15. Johnson, R., Quotient rings of rings with zero singular ideal, Pacific J. Math. 11 (1961), 1385–1392.Google Scholar
16. Kurshan, R., Rings whose cyclic modules have finitely generated socle, J. Algebra 15 (1970), 376–386.Google Scholar
17. Lambek, J., Lectures on rings and modules (Blaisdell Publishing Co., Waltham Mass., 1966).Google Scholar
18. Lambek, J., Torsion theories, additive semantics and rings of quotients, Lecture Notes in Mathematics No. 177 (Springer Verlag, Berlin and New York, 1971).Google Scholar
19. Morita, K., Duality for modules and its application to the theory of rings with minimum condition, Science Reports Tokyo Kyoiku Daigaku, 6 Ser. A, 1958.Google Scholar
20. Ornstein, A., Ph. D. Thesis, Rutgers University, 1967.Google Scholar
21. Osofsky, B., On twisted polynomial rings, J. Algebra 18 (1971), 405–414.Google Scholar
22. Sandomierski, F., Semisimple maximal quotient rings, Trans. Amer. Math. Soc. 128 (1967), 112–120.Google Scholar
23. Teply, M., Homological dimension and splitting torsion theories, Pacific J. Math. 34 (1970), 193–205.Google Scholar
24. Teply, M. and Fuelberth, D., The torsion submodule splits off, Math. Ann. 188 (1970), 270–284.Google Scholar
25. Utumi, Y., On quotient rings, Osaka Math. J. 8 (1956), 1–18.Google Scholar
26. Zelmanowitz, J., Endomorphism rings of torsionless modules, J. Algebra 5 (1967), 325–341.Google Scholar