Hostname: page-component-6bf8c574d5-r8w4l Total loading time: 0 Render date: 2025-03-07T02:25:02.308Z Has data issue: false hasContentIssue false
  • English
  • Français

Zero Tracts of Blaschke Products

Published online by Cambridge University Press:  20 November 2018

C. N. Linden  and
H. Somadasa
C. N. Linden
Affiliation:
University College of Swansea, Wales
H. Somadasa
Affiliation:
University College of Swansea, Wales
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let ﹛an﹜ be a sequence of complex numbers such that

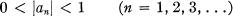
and

Then {an} is called a Blaschke sequence. For each Blaschke sequence {an} a Blaschke product is defined as

Thus a Blaschke product B(z, ﹛an﹜) is a function regular in the open unit disk D = {z: |z| < 1﹜ and having a zero at each point of the sequence ﹛an﹜.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 18 , 1966 , pp. 1072 - 1078
Copyright
Copyright © Canadian Mathematical Society 1966

References

1. Dienes, P., The Taylor series (New York, 1957).Google Scholar
2. Frostman, O., Potentiel d'équilibre et capacité des ensembles avec quelques applications à la théorie des fonctions (Lund, 1935).Google Scholar
3. Somadasa, H., Blaschke products with zero tangential limits, J. Lond. Math. Soc, 41 (1965), 293–303.Google Scholar