Hostname: page-component-745bb68f8f-b6zl4 Total loading time: 0 Render date: 2025-02-12T06:55:33.418Z Has data issue: false hasContentIssue false
  • English
  • Français

A function field analog of Jacobi’s theorem on sums of squares and its moments

Part of: Multiplicative number theory Finite fields and commutative rings (number-theoretic aspects)

Published online by Cambridge University Press:  10 January 2025

Wentang Kuo ,
Yu-Ru Liu  and
Yash Totani
Wentang Kuo
Affiliation:
Department of Pure Mathematics, University of Waterloo, Waterloo, ON, N2L3G1, Canada e-mail: wtkuo@uwaterloo.ca yrliu@uwaterloo.ca
Yu-Ru Liu
Affiliation:
Department of Pure Mathematics, University of Waterloo, Waterloo, ON, N2L3G1, Canada e-mail: wtkuo@uwaterloo.ca yrliu@uwaterloo.ca
Yash Totani*
Affiliation:
Department of Pure Mathematics, University of Waterloo, Waterloo, ON, N2L3G1, Canada e-mail: wtkuo@uwaterloo.ca yrliu@uwaterloo.ca
*
e-mail: ytotani@uwaterloo.ca
Get access Rights & Permissions [Opens in a new window]

Abstract

In this article, we establish a function field analog of Jacobi’s theorem on sums of squares and analyze its moments. Our approach involves employing two distinct techniques to derive the main results concerning asymptotic formulas for the moments. The first technique utilizes Dirichlet series framework to derive asymptotic formulas in the limit of large finite fields, specifically when the characteristic of 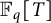$\mathbb {F}_q[T]$ becomes large. The second technique involves effectively partitioning the set of polynomials of a fixed degree, providing asymptotic formulas in the limit of large polynomial degree.

Keywords

Sums of squaresquadratic formsJacobi’s theoremnumber of representationspolynomial rings

MSC classification

Primary: 11T55: Arithmetic theory of polynomial rings over finite fields 11N37: Asymptotic results on arithmetic functions
Type
Article
Information
Canadian Mathematical Bulletin , First View , pp. 1 - 17
Check for updates
Copyright
© The Author(s), 2025. Published by Cambridge University Press on behalf of Canadian Mathematical Society

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bary-Soroker, L., Smilansky, Y., and Wolf, A., On the function field analogue of Landau’s theorem on sums of squares . Finite Fields Appl. 39(2016), 195–215.Google Scholar
Borwein, J. M. and Choi, K. S., On Dirichlet series for sums of squares . Ramanujan J. 7(2003), 95–127.Google Scholar
Connors, R. D. and Keating, J. P., Degeneracy moments for the square billiard . J. Phys. A 32(1999), 555–562.Google Scholar
Hardy, G. H. and Wright, E. M., An introduction to the theory of numbers, Oxford University Press, Oxford, 2008.Google Scholar
Karlin, S. and McGregor, J., Addendum to a paper of W. Ewens . Theoret. Popul. Biol. 3(1972), 113–116.Google Scholar
Landau, E., Vorlesungen über Zahlentheorie, Chelsea Publishing Co., New York, 1969.Google Scholar
Rosen, M., Number theory in function fields, Springer-Verlag, New York, 2002.Google Scholar