Article contents
Generalized 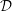 ${\mathcal{D}}$-Einstein Real Hypersurfaces in
${\mathcal{D}}$-Einstein Real Hypersurfaces in 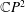 $\mathbb{C}P^{2}$ and
$\mathbb{C}P^{2}$ and 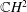 $\mathbb{C}H^{2}$
$\mathbb{C}H^{2}$
Published online by Cambridge University Press: 27 February 2020
Abstract
In this paper we obtain some new characterizations of pseudo-Einstein real hypersurfaces in 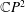 $\mathbb{C}P^{2}$ and
$\mathbb{C}P^{2}$ and 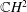 $\mathbb{C}H^{2}$. More precisely, we prove that a real hypersurface in
$\mathbb{C}H^{2}$. More precisely, we prove that a real hypersurface in 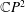 $\mathbb{C}P^{2}$ or
$\mathbb{C}P^{2}$ or 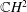 $\mathbb{C}H^{2}$ with constant mean curvature is generalized
$\mathbb{C}H^{2}$ with constant mean curvature is generalized  ${\mathcal{D}}$-Einstein with constant coefficient if and only if it is pseudo-Einstein. We prove that a real hypersurface in
${\mathcal{D}}$-Einstein with constant coefficient if and only if it is pseudo-Einstein. We prove that a real hypersurface in  $\mathbb{C}P^{2}$ with constant scalar curvature is generalized
$\mathbb{C}P^{2}$ with constant scalar curvature is generalized 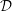 ${\mathcal{D}}$-Einstein with constant coefficient if and only if it is pseudo-Einstein.
${\mathcal{D}}$-Einstein with constant coefficient if and only if it is pseudo-Einstein.
MSC classification
- Type
- Article
- Information
- Copyright
- © Canadian Mathematical Society 2020
Footnotes
This work was supported by the Key Scientific Research Program in Universities of Henan Province (No. 20A110023) and the Fostering Foundation of National Foundation in Henan Normal University (No. 2019PL22).
References
- 4
- Cited by



