Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Jespers, E.
and
Leal, G.
1995.
Degree 1 and 2 representations of nilpotent groups and applications to units of group rings.
Manuscripta Mathematica,
Vol. 86,
Issue. 1,
p.
479.
Parmenter, M. M.
1995.
Conjugates of units in integral group rings.
Communications in Algebra,
Vol. 23,
Issue. 14,
p.
5503.
Jespers, Eric
and
Milies, C.Polcino
1996.
Units of group rings.
Journal of Pure and Applied Algebra,
Vol. 107,
Issue. 2-3,
p.
233.
Li, Yuanlin
1998.
Units OF Z(G × C2).
Quaestiones Mathematicae,
Vol. 21,
Issue. 3-4,
p.
201.
Sehgal, S.K.
2003.
Vol. 3,
Issue. ,
p.
455.
DOOMS, ANN
2006.
UNITARY UNITS IN INTEGRAL GROUP RINGS.
Journal of Algebra and Its Applications,
Vol. 05,
Issue. 01,
p.
43.
LOW, RICHARD M.
2008.
ON THE UNITS OF THE INTEGRAL GROUP RING Z[G × Cp].
Journal of Algebra and Its Applications,
Vol. 07,
Issue. 03,
p.
393.
Bilgin, Tevfik
Kusmus, Omer
and
Low, Richard M.
2016.
A CHARACTERIZATION OF THE UNIT GROUP IN ℤ[T×C2].
Bulletin of the Korean Mathematical Society,
Vol. 53,
Issue. 4,
p.
1105.
Bächle, Andreas
Maheshwary, Sugandha
and
Margolis, Leo
2021.
Abelianization of the unit group of an integral group ring.
Pacific Journal of Mathematics,
Vol. 312,
Issue. 2,
p.
309.
Malik, Sandeep
and
Sharma, R. K.
2024.
Describing the group of units of integral grouprings ℤD8 and ℤD12.
Asian-European Journal of Mathematics,
Vol. 17,
Issue. 01,
Janssens, Geoffrey
Jespers, Eric
and
Schnabel, Ofir
2024.
Units of twisted group rings and their correlations to classical group rings.
Advances in Mathematics,
Vol. 458,
Issue. ,
p.
109983.
Küsmüş, Ömer
2024.
Unit group of integral group ring ℤ(G × C3).
Miskolc Mathematical Notes,
Vol. 25,
Issue. 2,
p.
845.
Küsmüş, Ömer
Denizler, İsmail
and
Low, Richard M.
2024.
On the Unit Group of the Integral Group Ring Z(S_3×C_3).
Yüzüncü Yıl Üniversitesi Fen Bilimleri Enstitüsü Dergisi,
Vol. 29,
Issue. 1,
p.
157.
Sharma, R. K.
and
Gupta, Abhinay Kumar
2025.
Characterization of the unit group of ℤ[G × C2n].
Journal of Algebra and Its Applications,
Vol. 24,
Issue. 02,


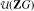 for the two groups
for the two groups 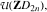 for all n ≥ 3.
for all n ≥ 3.