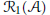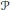Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Tat Thang, Nguyen
2014.
Admissibility of Local Systems for some Classes of Line Arrangements.
Canadian Mathematical Bulletin,
Vol. 57,
Issue. 3,
p.
658.
Bailet, Pauline
2014.
On the Monodromy of Milnor Fibers of Hyperplane Arrangements.
Canadian Mathematical Bulletin,
Vol. 57,
Issue. 4,
p.
697.