Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Dubsky, Brendan Frisk
Guo, Xiangqian
Yao, Yufeng
and
Zhao, Kaiming
2019.
Simple modules over the Lie algebras of divergence zero vector fields on a torus.
Forum Mathematicum,
Vol. 31,
Issue. 3,
p.
727.
Bavula, V. V.
2020.
Classification of Simple Modules of the Ore Extension $$K[X][Y; f\frac{d}{dX}]$$.
Mathematics in Computer Science,
Vol. 14,
Issue. 2,
p.
317.
Bavula, Volodymyr
and
Lu, Tao
2021.
The prime spectrum of the universal enveloping algebra of the 1-spatial ageing algebra and of U(gl2).
Algebra and Discrete Mathematics,
Vol. 31,
Issue. 1,
p.
1.
Tao, W.-Q.
2021.
On representations of the centrally extended Heisenberg double of SL2.
Journal of Mathematical Physics,
Vol. 62,
Issue. 7,
MAZORCHUK, VOLODYMYR
and
MRÐEN, RAFAEL
2022.
LIE ALGEBRA MODULES WHICH ARE LOCALLY FINITE AND WITH FINITE MULTIPLICITIES OVER THE SEMISIMPLE PART.
Nagoya Mathematical Journal,
Vol. 246,
Issue. ,
p.
430.
Cai, Yan-an
and
Yan, Huimin
2022.
Electrical Lie algebras, the Schrödinger algebras and their representations.
Journal of Geometry and Physics,
Vol. 181,
Issue. ,
p.
104646.
Tao, W.-Q.
2022.
On simple modules of the n-th Schrödinger algebra.
Journal of Pure and Applied Algebra,
Vol. 226,
Issue. 5,
p.
106944.
Cai, Yan-an
and
Liu, Zedong
2023.
Whittaker modules and quasi-Whittaker modules for the Schrödinger algebra in (2 + 1)-dimensional spacetime.
Journal of Geometry and Physics,
Vol. 186,
Issue. ,
p.
104769.
Chen, Zhengxin
and
Wang, Yu
2023.
Quasi-Whittaker modules for the n-th Schrödinger algebra.
Linear Algebra and its Applications,
Vol. 677,
Issue. ,
p.
51.
Cai, Yan-an
He, Yan
and
Lü, Rencai
2023.
Module structures on U(S−) for the Schrödinger algebra.
Journal of Geometry and Physics,
Vol. 191,
Issue. ,
p.
104919.
Chen, Zhengxin
and
Wang, Yu
2023.
Zero product determined n-th Schrödinger algebra.
Linear Algebra and its Applications,
Vol. 679,
Issue. ,
p.
165.
Chen, Zhengxin
and
Wang, Yu
2023.
Derivations and biderivations of the n-th Schrödinger algebra.
Communications in Algebra,
Vol. 51,
Issue. 3,
p.
1049.
Chen, Zhengxin
and
Wang, Yu
2024.
U
(h)-free modules on
n
-th Schrödinger algebra
.
Communications in Algebra,
Vol. 52,
Issue. 3,
p.
1283.
Lei, Boqing
and
Yang, Hengyun
2024.
The derivation algebra and automorphism group of the
n
-th Schrödinger algebra
.
Communications in Algebra,
Vol. 52,
Issue. 1,
p.
283.
Tao, Wenqing
2025.
The Coupled q-oscillator Algebra and a Classification of Its Simple Weight Modules.
Frontiers of Mathematics,
He, Yan
and
Wang, Xinyue
2025.
Module structures on U(S−) for the super Schrödinger algebra.
Communications in Algebra,
p.
1.
Lu, Tao
2025.
On simple weight modules of the centrally extended Heisenberg double of quantum SL
2
.
Communications in Algebra,
p.
1.
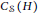 ${{C}_{S}}(H)$ (and some of its prime factor algebras) of the Cartan element
${{C}_{S}}(H)$ (and some of its prime factor algebras) of the Cartan element  $H$ in the universal enveloping algebra
$H$ in the universal enveloping algebra 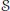 $S$ of the Schrödinger (Lie) algebra. The simple
$S$ of the Schrödinger (Lie) algebra. The simple 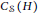 ${{C}_{S}}(H)$-modules are classified. The Krull and the global dimensions are found for some (prime) factor algebras of the algebra
${{C}_{S}}(H)$-modules are classified. The Krull and the global dimensions are found for some (prime) factor algebras of the algebra 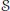 $S$ (over the centre). It is proved that some (prime) factor algebras of
$S$ (over the centre). It is proved that some (prime) factor algebras of 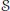 $S$ and
$S$ and 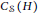 ${{C}_{S}}(H)$ are tensor homological
${{C}_{S}}(H)$ are tensor homological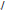 $/$Krull minimal.
$/$Krull minimal.

