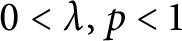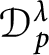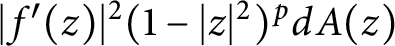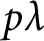Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Xie, Huayou
Lin, Qingze
and
Liu, Junming
2024.
Cesàro-type operators on Bergman–Morrey spaces and Dirichlet–Morrey spaces.
Proceedings of the Edinburgh Mathematical Society,
p.
1.