Hostname: page-component-78c5997874-94fs2 Total loading time: 0 Render date: 2024-11-15T09:31:06.702Z Has data issue: false hasContentIssue false
  • English
  • Français

A Counterexample to a Conjecture of D. B. Fuks

Published online by Cambridge University Press:  20 November 2018

Luc Demers
Luc Demers*
Affiliation:
University of Ottawa, Ottawa, Ontario
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

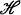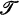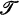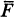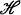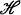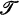In [3] D. B. Fuks defined a duality of functors in the category of weak homotopy types. In general this duality is more difficult to work with than the duality of functors of the category of pointed Kelley spaces [2]. It happens however that all so-called strong functors [2] F of induce functors of , and if we denote the duality operators of and by 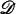and D respectively, then there are many cases where .

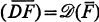Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 13 , Issue 2 , June 1970 , pp. 261 - 266
Copyright
Copyright © Canadian Mathematical Society 1970

References

1. Bott, R. and Samelson, H., On the Pontryagin product in spaces of paths, Comment. Math. Helv. 27 (1953), 320-337.Google Scholar
2. Fuks, D. B., Eckmann- Hilton duality and the theory of functors in the category of topological spaces, Russian Math. Surveys (2) 21 (1966), 1-33.Google Scholar
3. Fuks, D. B., Duality of functors in the category ofhomotopy types, Soviet Math. Dokl. 8 (1967), 1007-1010.Google Scholar
4. Hilton, P. J., Homotopy theory and duality, Gordon and Breach, New York, 1965.Google Scholar