Published online by Cambridge University Press: 20 November 2018
Soient 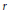 $r$ et
$r$ et  $p$ deux nombres premiers distincts, soit
$p$ deux nombres premiers distincts, soit
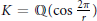 $K\,=\,\mathbb{Q}(\cos \,\frac{2\pi }{r})$
, et soit
$K\,=\,\mathbb{Q}(\cos \,\frac{2\pi }{r})$
, et soit 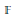 $\mathbb{F}$ le corps résiduel de
$\mathbb{F}$ le corps résiduel de 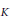 $K$ en une place au-dessus de
$K$ en une place au-dessus de  $p$. Lorsque l’image de
$p$. Lorsque l’image de
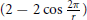 $(2\,-\,2\,\cos \,\frac{2\pi }{r})$
dans
$(2\,-\,2\,\cos \,\frac{2\pi }{r})$
dans 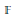 $\mathbb{F}$ n’est pas un carré, nous donnons une construction géométrique d’une extension réguliere de
$\mathbb{F}$ n’est pas un carré, nous donnons une construction géométrique d’une extension réguliere de 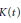 $K\left( t \right)$ de groupe de Galois
$K\left( t \right)$ de groupe de Galois
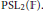 $\text{PS}{{\text{L}}_{2}}(\mathbb{F})$
. Cette extension correspond à un revêtement de
$\text{PS}{{\text{L}}_{2}}(\mathbb{F})$
. Cette extension correspond à un revêtement de
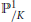 ${{\mathbb{P}}^{1}}/k$
de « signature
${{\mathbb{P}}^{1}}/k$
de « signature 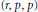 $\left( r,\,p,\,p \right)$ » au sens de [3, sec. 6.3], et son existence est prédite par le critère de rigidité de Belyi, Fried, Thompson et Matzat. Sa construction s’obtient en tordant la representation galoisienne associée aux points d’ordre
$\left( r,\,p,\,p \right)$ » au sens de [3, sec. 6.3], et son existence est prédite par le critère de rigidité de Belyi, Fried, Thompson et Matzat. Sa construction s’obtient en tordant la representation galoisienne associée aux points d’ordre  $p$ d’une famille de variétés abéliennes à multiplications réelles par
$p$ d’une famille de variétés abéliennes à multiplications réelles par 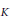 $K$ découverte par Tautz, Top et Verberkmoes [6]. Ces variétés abéliennes sont définies sur un corps quadratique, et sont isogènes à leur conjugué galoisien. Notre construction généralise une méthode de Shih [4], [5], que l’on retrouve quand
$K$ découverte par Tautz, Top et Verberkmoes [6]. Ces variétés abéliennes sont définies sur un corps quadratique, et sont isogènes à leur conjugué galoisien. Notre construction généralise une méthode de Shih [4], [5], que l’on retrouve quand 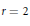 $r\,=\,2$ et
$r\,=\,2$ et 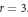 $r\,=\,3$.
$r\,=\,3$.
Let 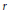 $r$ and
$r$ and  $p$ be distinct prime numbers, let
$p$ be distinct prime numbers, let
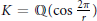 $K\,=\,\mathbb{Q}(\cos \,\frac{2\pi }{r})$
, and let
$K\,=\,\mathbb{Q}(\cos \,\frac{2\pi }{r})$
, and let 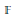 $\mathbb{F}$ be the residue field of
$\mathbb{F}$ be the residue field of 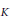 $K$ at a place above
$K$ at a place above  $p$. When the image of
$p$. When the image of
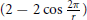 $(2\,-\,2\,\cos \,\frac{2\pi }{r})$
in
$(2\,-\,2\,\cos \,\frac{2\pi }{r})$
in 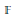 $\mathbb{F}$ is not a square, we describe a geometric construction of a regular extension of
$\mathbb{F}$ is not a square, we describe a geometric construction of a regular extension of 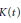 $K\left( t \right)$ with Galois group
$K\left( t \right)$ with Galois group
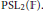 $\text{PS}{{\text{L}}_{2}}(\mathbb{F})$
. This extension corresponds to a covering of
$\text{PS}{{\text{L}}_{2}}(\mathbb{F})$
. This extension corresponds to a covering of
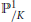 ${{\mathbb{P}}^{1}}/k$
of “signature
${{\mathbb{P}}^{1}}/k$
of “signature 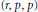 $\left( r,\,p,\,p \right)$” in the sense of [3, sec. 6.3], and its existence is predicted by the rigidity criterion of Belyi, Fried, Thompson and Matzat. Its construction is obtained by twisting the mod
$\left( r,\,p,\,p \right)$” in the sense of [3, sec. 6.3], and its existence is predicted by the rigidity criterion of Belyi, Fried, Thompson and Matzat. Its construction is obtained by twisting the mod  $p$ galois representation attached to a family of abelian varieties with real multiplications by
$p$ galois representation attached to a family of abelian varieties with real multiplications by 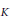 $K$ discovered by Tautz, Top and Verberkmoes [6]. These abelian varieties are defined in general over a quadratic field, and are isogenous to their galois conjugate. Our construction generalises a method of Shih [4], [5], which one recovers when
$K$ discovered by Tautz, Top and Verberkmoes [6]. These abelian varieties are defined in general over a quadratic field, and are isogenous to their galois conjugate. Our construction generalises a method of Shih [4], [5], which one recovers when 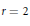 $r\,=\,2$ and
$r\,=\,2$ and 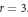 $r\,=\,3$.
$r\,=\,3$.