Hostname: page-component-7bb8b95d7b-495rp Total loading time: 0 Render date: 2024-09-20T14:49:17.335Z Has data issue: false hasContentIssue false
  • English
  • Français

Degenerations of Leibniz and Anticommutative Algebras

Part of: Families, fibrations General nonassociative rings Algebraic groups

Published online by Cambridge University Press:  29 January 2019

Nurlan Ismailov ,
Ivan Kaygorodov  and
Yury Volkov
Nurlan Ismailov
Affiliation:
Universidade de São Paulo, IME, São Paulo, Brazil Institute of Mathematics and Mathematical Modeling, Almaty, Kazakhstan Email: nurlan.ismail@gmail.com
Ivan Kaygorodov
Affiliation:
Universidade Federal do ABCCMCC, Santo André, Brazil Email: kaygorodov.ivan@gmail.com
Yury Volkov
Affiliation:
Saint Petersburg State University, Saint Petersburg, Russia Email: wolf86_666@list.ru
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We describe all degenerations of three-dimensional anticommutative algebras $\mathfrak{A}\mathfrak{c}\mathfrak{o}\mathfrak{m}_{3}$ and of three-dimensional Leibniz algebras 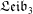$\mathfrak{L}\mathfrak{e}\mathfrak{i}\mathfrak{b}_{3}$ over 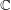$\mathbb{C}$. In particular, we describe all irreducible components and rigid algebras in the corresponding varieties.

Keywords

degenerationrigid algebraorbit closureanticommutative algebraLeibniz algebraLie algebra

MSC classification

Primary: 17A32: Leibniz algebras
Secondary: 14D06: Fibrations, degenerations 14L30: Group actions on varieties or schemes (quotients)
Type
Article
Information
Canadian Mathematical Bulletin , Volume 62 , Issue 3 , September 2019 , pp. 539 - 549
Copyright
© Canadian Mathematical Society 2019 

Footnotes

The work was supported by FAPESP 17/15437-6, 17/21429-6; AP05131123 “Cohomological and structural problems of non-associative algebras”; RFBR 18-31-00001; the President’s Program “Support of Young Russian Scientists” (grant MK-2262.2019.1).

References

Alvarez, M. A., On rigid 2-step nilpotent Lie algebras . Algebra Colloq. 25(2018), 2, 349–360. https://doi.org/10.1142/S100538671800024X.Google Scholar
Alvarez, M. A., The variety of 7-dimensional 2-step nilpotent Lie algebras . Symmetry 10(2018), 1, 26. https://doi.org/10.3390/sym10010026.Google Scholar
Alvarez, M. A. and Hernández I., I., On degenerations of Lie superalgebras . Linear Multilinear Algebra, to appear. https://doi.org/10.1080/03081087.2018.1498060.Google Scholar
Alvarez, M. A., Hernández, I., and Kaygorodov, I., Degenerations of Jordan superalgebras . Bull. Malays. Math. Sci. Soc. 43(2020), https://doi.org/10.1007/s40840-018-0664-3.Google Scholar
Bekbaev, U., Complete classification of a class of m-dimensional algebras . J. Phys. Conf. Ser. 819(2017), 012012. https://doi.org/10.1088/1742-6596/819/1/012012.Google Scholar
Benes, T. and Burde, D., Degenerations of pre-Lie algebras . J. Math. Phys. 50(2009), 11, 112102. https://doi.org/10.1063/1.3246608.Google Scholar
Benes, T. and Burde, D., Classification of orbit closures in the variety of three dimensional Novikov algebras . J. Algebra Appl. 13(2014), 2, 1350081. https://doi.org/10.1142/S0219498813500813.Google Scholar
Burde, D., Degenerations of nilpotent Lie algebras . J. Lie Theory 9(1999), 1, 193–202.Google Scholar
Burde, D. and Steinhoff, C., Classification of orbit closures of 4-dimensional complex Lie algebras . J. Algebra 214(1999), 2, 729–739. https://doi.org/10.1006/jabr.1998.7714.Google Scholar
Calderón, A., Fernández Ouaridi, A., and Kaygorodov, I., The classification of n-dimensional anticommutative algebras with (n - 3)-dimensional annihilator . Comm. Algebra 47(2019), 1, 173–181. https://doi.org/10.1080/00927872.2018.1468909.Google Scholar
Casas, J., Insua, M., Ladra, M., and Ladra, S., An algorithm for the classification of 3-dimensional complex Leibniz algebras . Linear Algebra Appl. 436(2012), 9, 3747–3756. https://doi.org/10.1016/j.laa.2011.11.039.Google Scholar
Casas, J., Khudoyberdiyev, A., Ladra, M., and Omirov, B., On the degenerations of solvable Leibniz algebras . Linear Algebra Appl. 439(2013), 2, 472–487. https://doi.org/10.1016/j.laa.2013.03.029.Google Scholar
Fialowski, A. and Penkava, M., The moduli space of 4-dimensional nilpotent complex associative algebras . Linear Algebra Appl. 457(2014), 408–427. https://doi.org/10.1016/j.laa.2014.05.014.Google Scholar
Gainov, A. T., Binary Lie algebras of lower ranks. (Russian) . Algebra i Logika Sem. 2(1963), 4, 21–40.Google Scholar
Grunewald, F. and O’Halloran, J., Varieties of nilpotent Lie algebras of dimension less than six . J. Algebra 112(1988), 315–325. https://doi.org/10.1016/0021-8693(88)90093-2.Google Scholar
Grunewald, F. and O’Halloran, J., A characterization of orbit closure and applications . J. Algebra 116(1988), 163–175. https://doi.org/10.1016/0021-8693(88)90199-8.Google Scholar
Grunewald, F. and O’Halloran, J., Deformations of Lie algebras . J. Algebra 162(1993), 1, 210–224. https://doi.org/10.1006/jabr.1993.1250.Google Scholar
Horn, R. A. and Sergeichuk, V., Canonical matrices of bilinear and sesquilinear forms . Linear Algebra Appl. 428(2008), 1, 193–223. https://doi.org/10.1016/j.laa.2007.07.023.Google Scholar
Ismailov, N., Kaygorodov, I., and Volkov, Yu., The geometric classification of Leibniz algebras . Internat. J. Math. 29(2018), 5, 1850035. https://doi.org/10.1142/S0129167X18500350.Google Scholar
Kaygorodov, I., Popov, Yu., and Volkov, Yu., Degenerations of binary Lie and nilpotent Malcev algebras . Comm. Algebra 46(2018), 11, 4929–4940. https://doi.org/10.1080/00927872.2018.1459647.Google Scholar
Kaygorodov, I., Popov, Yu., Pozhidaev, A., and Volkov, Yu., Degenerations of Zinbiel and nilpotent Leibniz algebras . Linear Multilinear Algebra 66(2018), 4, 704–716. https://doi.org/10.1080/03081087.2017.1319457.Google Scholar
Kaygorodov, I. and Volkov, Yu., The variety of 2-dimensional algebras over an algebraically closed field . Canad. J. Math., to appear. https://doi.org/10.4153/S0008414X18000056.Google Scholar
Kaygorodov, I. and Volkov, Yu., Complete classification of algebras of level two . Moscow Math. J., to appear. arxiv:1710.08943.Google Scholar
Kobayashi, Yu., Shirayanagi, K., Takahasi, S., and Tsukada, M., Classification of three dimensional zeropotent algebras over an algebraically closed field . Comm. Algebra 45(2017), 12, 5037–5052. https://doi.org/10.1080/00927872.2017.1313426.Google Scholar
Khudoyberdiyev, A. and Omirov, B., The classification of algebras of level one . Linear Algebra Appl. 439(2013), 11, 3460–3463. https://doi.org/10.1016/j.laa.2013.09.020.Google Scholar
Khudoyberdiyev, A., Ladra, M., Masutova, K., and Omirov, B., Some irreducible components of the variety of complex (n + 1)-dimensional Leibniz algebras . J. Geom. Phys. 121(2017), 228–246. https://doi.org/10.1016/j.geomphys.2017.07.014.Google Scholar
Lauret, J., Degenerations of Lie algebras and geometry of Lie groups . Differ. Geom. Appl. 18(2003), 2, 177–194. https://doi.org/10.1016/S0926-2245(02)00146-8.Google Scholar
Loday, J.-L. and Pirashvili, T., Universal enveloping algebras of Leibniz algebras and (co)homology . Math. Ann. 296(1993), 1, 139–158. https://doi.org/10.1007/BF01445099.Google Scholar
Rakhimov, I. and Mohd Atan, K., On contractions and invariants of Leibniz algebras . Bull. Malays. Math. Sci. Soc. 35(2012), 557–565.Google Scholar
Seeley, C., Degenerations of 6-dimensional nilpotent Lie algebras over ℂ . Comm. Algebra 18(1990), 3493–3505. https://doi.org/10.1080/00927879008824088.Google Scholar