Published online by Cambridge University Press: 20 November 2018
Let n ∊ ℤ+ and R be a ring which possesses a unit element, a left ideal J, and a derivation d such that dn(J) ≠ 0 and dn(r) is 0 or invertible, for all r ∊ J. We prove that either R is primitive, in which case R is Di with 1 ≤ i ≤ n+ 1, where Di is the ring of i × i matrices over a division ring D, or else there exist positive integers i, l and p with p prime and 2 ≤ ipl ≤ n + 1, such that R is 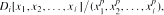 where D is a division ring with characteristic p, and furthermore there is a derivation f of Di and a1, a2,..,al ∊ ZDi., the center of Di, such that a ∊ Di then
where D is a division ring with characteristic p, and furthermore there is a derivation f of Di and a1, a2,..,al ∊ ZDi., the center of Di, such that a ∊ Di then

and

for all 2 ≤ j ≤ l