Hostname: page-component-78c5997874-94fs2 Total loading time: 0 Render date: 2024-11-10T11:55:26.719Z Has data issue: false hasContentIssue false
  • English
  • Français

Dyson’s rank, overpartitions, and universal mock theta functions

Part of: Additive number theory; partitions Sequences and sets Discontinuous groups and automorphic forms

Published online by Cambridge University Press:  07 September 2020

Helen W. J. Zhang
Helen W. J. Zhang*
Affiliation:
School of Mathematics, Hunan University, Changsha410082, People's Republic of China
*
e-mail: helenzhang@hnu.edu.cn
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper, we decompose 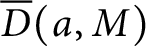$\overline {D}(a,M)$ into modular and mock modular parts, so that it gives as a straightforward consequencethe celebrated results of Bringmann and Lovejoy on Maass forms. Let 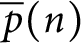$\overline {p}(n)$ be the number of partitions of n and 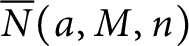$\overline {N}(a,M,n)$ be the number of overpartitions of n with rank congruent to a modulo M. Motivated by Hickerson and Mortenson, we find and prove a general formula for Dyson’s ranks by considering the deviation of the ranks from the average:

$$ \begin{align*} \overline{D}(a,M) &=\sum\limits_{n=0}^{\infty}\Big(\overline{N}(a,M,n) -\frac{\overline{p}(n)}{M}\Big)q^{n}. \end{align*} $$
Based on Appell–Lerch sum properties and universal mock theta functions, we obtain the stronger version of the results of Bringmann and Lovejoy.

Keywords

OverpartitionDyson’s rankAppell-Lerch sumuniversal mock theta functions

MSC classification

Primary: 11P84: Partition identities; identities of Rogers-Ramanujan type
Secondary: 11B65: Binomial coefficients; factorials; $q$-identities 11F11: Holomorphic modular forms of integral weight 11F27: Theta series; Weil representation; theta correspondences
Type
Article
Information
Canadian Mathematical Bulletin , Volume 64 , Issue 3 , September 2021 , pp. 687 - 696
Check for updates
Copyright
© Canadian Mathematical Society 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The author would like to thank the referees for their valuable comments and suggestions. This work was supported by the National Science Foundation of China (Grant Nos. 11871370 and 12001182) and the Fundamental Research Funds for the Central Universities (Grant No. 531118010411).

References

Andrews, G. E. and Garvan, F. G., Ramanujan’s “lost” notebook VI: The mock theta conjectures. Adv. Math. 73(1989), 245–255. http://dx.doi.org/10.1016/0001-8708(89)90070-4 CrossRefGoogle Scholar
Atkin, A. O. L. and Swinnerton-Dyer, P., Some properties of partitions. Proc. Lond. Math. Soc. 4(1954), 84–106. http://dx.doi.org/10.1112/plms/s3-4.1.84 CrossRefGoogle Scholar
Bringmann, K. and Ono, K., The $f(q)$ mock theta function conjecture and partition ranks. Invent. Math. 165(2006), 243–266. http://dx.doi.org/10.1007/s00222-005-0493-5 CrossRefGoogle Scholar
Bringmann, K. and Ono, K., Dyson’s ranks and Maass forms. Ann. of Math. (2) 171(2010), 419–449. http://dx.doi.org/10.4007/annals.2010.171.419 CrossRefGoogle Scholar
Bringmann, K. and Lovejoy, J., Dyson’s rank, overpartitions, and weak Maass forms. Int. Math. Res. Not. 2007(2007), no. 19, rnm063. http://dx.doi.org/10.1093/imrn/rnm063 Google Scholar
Choi, Y.-S., Tenth order mock theta functions in Ramanujan’s lost notebook. Invent. Math. 136(1999), 497–569. http://dx.doi.org/10.1007/s002220050318 CrossRefGoogle Scholar
Corteel, S. and Lovejoy, J., Overpartitions. Trans. Amer. Math. Soc. 356(2004) 1623–1635. http://dx.doi.org/10.1090/S0002-9947-03-03328-2 CrossRefGoogle Scholar
Dyson, F. J., Some guesses in the theory of partitions . Eureka 8(1944), 10–15.Google Scholar
Gordon, B. and McIntosh, R. J., A survey of classical mock theta functions. In: Partitions, $q$ -series, and modular forms, Dev. Math. 23, Springer, New York, 2012, pp. 95–144. http://dx.doi.org/10.1007/978-1-4614-0028-8_9 Google Scholar
Hickerson, D., A proof of the mock theta conjectures. Invent. Math. 94(1988), 639–660. http://dx.doi.org/10.1007/BF01394279 CrossRefGoogle Scholar
Hickerson, D., On the seventh order mock theta functions. Invent. Math. 94(1988), 661–677. http://dx.doi.org/10.1007/BF01394280 CrossRefGoogle Scholar
Hickerson, D. and Mortenson, E., Hecke-type double sums, Appell-Lerch sums, and mock theta functions I. Proc. Lond. Math. Soc. (3) 109(2014), 382–422. http://dx.doi.org/10.1112/plms/pdu007 CrossRefGoogle Scholar
Hickerson, D. and Mortenson, E., Dyson’s ranks and Appell-Lerch sums. Math. Ann. 367(2017), 373–395. http://dx.doi.org/10.1007/s00208-016-1390-5 CrossRefGoogle Scholar
Jennings-Shaffer, C., The generating function of the ${M}_2$ -rank of partitions without repeated odd parts as a mock modular form. Trans. Amer. Math. Soc. 371(2019), 249–277. http://dx.doi.org/10.1090/tran/7212 CrossRefGoogle Scholar
Lovejoy, J., Rank and conjugation for the Frobenius representation of an overpartition. Ann. Comb. 9(2005), 321–334. http://dx.doi.org/10.1007/s00026-005-0260-8 CrossRefGoogle Scholar
Lovejoy, J. and Osburn, R., Rank differences for overpartitions. Q. J. Math. 59(2008), 257–273. http://dx.doi.org/10.1093/qmath/ham031 CrossRefGoogle Scholar
Wei, B. and Zhang, H. W. J., Rank differences for overpartitions modulo $6$ . Proc. Amer. Math. Soc. 148(2020), no. 10, 4333–4349. http://dx.doi.org/10.1090/proc/15075 CrossRefGoogle Scholar
Zagier, D., Ramanujan’s mock theta functions and their applications. Séminaire Bourbaki. Vol. 2007/2008. Astérisque No. 326 (2009), Exp. No. 986, vii–viii, 143–164 (2010).Google Scholar