Article contents
Enumerating Unlabelled Embeddings of Digraphs
Published online by Cambridge University Press: 20 November 2018
Abstract
A 2-cell embedding of an Eulerian digraph 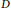 $D$ into a closed surface is said to be directed if the boundary of each face is a directed closed walk in
$D$ into a closed surface is said to be directed if the boundary of each face is a directed closed walk in 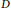 $D$. In this paper, a method is developed with the purpose of enumerating unlabelled embeddings for an Eulerian digraph. As an application, we obtain explicit formulas for the number of unlabelled embeddings of directed bouquets of cycles
$D$. In this paper, a method is developed with the purpose of enumerating unlabelled embeddings for an Eulerian digraph. As an application, we obtain explicit formulas for the number of unlabelled embeddings of directed bouquets of cycles 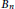 ${{B}_{n}}$, directed dipoles
${{B}_{n}}$, directed dipoles 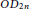 $O{{D}_{2n}}$ and for a class of regular tournaments
$O{{D}_{2n}}$ and for a class of regular tournaments 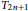 ${{T}_{2n+1}}$.
${{T}_{2n+1}}$.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2018
References
- 1
- Cited by


