Existence of hypercylinder expanders of the inverse mean curvature flow

Abstract

We will give a new proof of the existence of hypercylinder expander of the inverse mean curvature flow which is a radially symmetric homothetic soliton of the inverse mean curvature flow in 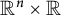$\mathbb {R}^{n}\times \mathbb {R}$ 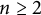, $n\ge 2$ , of the form $(r,y(r))$ or 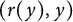$(r(y),y)$ , where 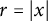$r=|x|$ 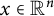, $x\in \mathbb {R}^{n}$ , is the radially symmetric coordinate and 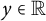$y\in \mathbb {R}$ . 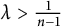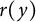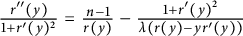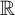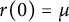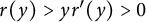More precisely, for any $\lambda>\frac {1}{n-1}$ and $\mu>0$ , we will give a new proof of the existence of a unique even solution $r(y)$ of the equation $\frac {r^{\prime \prime }(y)}{1+r^{\prime }(y)^{2}}=\frac {n-1}{r(y)}-\frac {1+r^{\prime }(y)^{2}}{\lambda (r(y)-yr^{\prime }(y))}$ in $\mathbb {R}$ which satisfies $r(0)=\mu $ , $r^{\prime }(0)=0$ and $r(y)>yr^{\prime }(y)>0$ for any $y\in \mathbb {R}$ . We will prove that 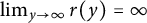$\lim _{y\to \infty }r(y)=\infty $ and $a_{1}:=\lim _{y\to \infty }r^{\prime }(y)$ exists with 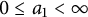$0\le a_{1}<\infty $ . We will also give a new proof of the existence of a constant 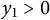$y_{1}>0$ such that 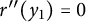$r^{\prime \prime }(y_{1})=0$ 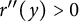, $r^{\prime \prime }(y)>0$ for any 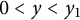$0<y<y_{1}$ , and 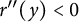$r^{\prime \prime }(y)<0$ for any 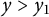$y>y_{1}$ .