Hostname: page-component-78c5997874-lj6df Total loading time: 0 Render date: 2024-11-15T04:28:53.606Z Has data issue: false hasContentIssue false
  • English
  • Français

A Generalization of a Theorem of Hilton

Published online by Cambridge University Press:  20 November 2018

C. S. Hoo
C. S. Hoo*
Affiliation:
University of Alberta Edmonton
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let f: A×B → X be a map. Let J(f): ∑(A ∧ B)→ ∑X be the map obtained from f by means of the Hopf construction. Let P(f) denote the space obtained from ∑X by attaching a cone on ∑(A ∧ B) by means of J(f). Let l: ∑X→P(f ) be the inclusion and T(l): X→ΩP(f) the adjoint of l. Let h1,: A1, → A, h2: B1,→ B be maps. Let c:ΩP(f)× ΩP(f) → ΩP(f) be the basic commutator. Then we prove that there exists a map ∑A1 × ∑B1 →P(f) with axes l∑(fi1 h1), l∑(fi2 h2) if and only if 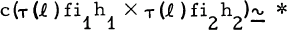, where i1: A→ A × B and i2 B → A × B are the inclusions. This generalizes a result of Hilton. Also, by letting f be an H-space multiplication and h and h the identity maps, we obtain a well known criterion of Stasheff for an H-space to be homotopy-commutative. Finally, appropriate duals of these results are given.

Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 11 , Issue 5 , December 1968 , pp. 663 - 669
Copyright
Copyright © Canadian Mathematical Society 1968

References

1. Arkowitz, M., The generalized Whitehead product,. Pac. J. Math. 12 (1962) 7-23.Google Scholar
2. Arkowitz, M., Homotopy products for H-spaces, Mich. Math. J. 10 (1963) 1-9.Google Scholar
3. Arkowitz, M., Commutators and cup products, 111. J. Math. 8 (1964) 571-581.Google Scholar
4. Berstein, I. and Ganea, T., Homotopical nilpotency, 111. J. Math. 5 (1961) 99-130.Google Scholar
5. Berstein, I. and Hilton, P. J., On suspensions and comultiplications, Topology 2 (1963) 73-82.Google Scholar
6. Hilton, P.J., Note on a theorem of Stasheff, Bull. Pol. Acad. Sci. 10 (1962) 127-131.Google Scholar
7. Hilton, P.J., Homotopy theory and duality (Gordon and Breach, New York, 1965).Google Scholar
8. Hoo, C. S., A note on a theorem of Ganea, Hilton and Peterson. Proc. Amer. Math. Soc. 19 (1968) 909-911.Google Scholar
9. Stasheff, J., On homotopy Abelian H-spaces, Proc. Camb. Phil. Soc. 57 (1961) 734-745.Google Scholar