Published online by Cambridge University Press: 20 November 2018
Let  $R$ be a ring and let
$R$ be a ring and let  $g$ be an endomorphism of
$g$ be an endomorphism of  $R$. The additive mapping
$R$. The additive mapping  $d:\,R\,\to \,R$ is called a Jordan semiderivation of
$d:\,R\,\to \,R$ is called a Jordan semiderivation of  $R$, associated with
$R$, associated with  $g$, if
$g$, if
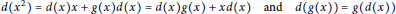 $$d\left( {{x}^{2}} \right)=d\left( x \right)x+g\left( x \right)d\left( x \right)=d\left( x \right)g\left( x \right)+xd\left( x \right)\,\text{and}\,d\left( g\left( x \right) \right)=g\left( d\left( x \right) \right)$$
$$d\left( {{x}^{2}} \right)=d\left( x \right)x+g\left( x \right)d\left( x \right)=d\left( x \right)g\left( x \right)+xd\left( x \right)\,\text{and}\,d\left( g\left( x \right) \right)=g\left( d\left( x \right) \right)$$
for all 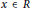 $x\,\in \,R$. The additive mapping
$x\,\in \,R$. The additive mapping 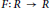 $F:\,R\,\to \,R$ is called a generalized Jordan semiderivation of
$F:\,R\,\to \,R$ is called a generalized Jordan semiderivation of  $R$, related to the Jordan semiderivation
$R$, related to the Jordan semiderivation  $d$ and endomorphism
$d$ and endomorphism  $g$, if
$g$, if
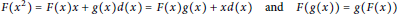 $$F\left( {{x}^{2}} \right)=F\left( x \right)x+g\left( x \right)d\left( x \right)=F\left( x \right)g\left( x \right)+xd\left( x \right)\,\,and\,F\left( g\left( x \right) \right)=g\left( F\left( x \right) \right)$$
$$F\left( {{x}^{2}} \right)=F\left( x \right)x+g\left( x \right)d\left( x \right)=F\left( x \right)g\left( x \right)+xd\left( x \right)\,\,and\,F\left( g\left( x \right) \right)=g\left( F\left( x \right) \right)$$
for all 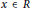 $x\,\in \,R$. In this paper we prove that if
$x\,\in \,R$. In this paper we prove that if  $R$ is a prime ring of characteristic different from 2,
$R$ is a prime ring of characteristic different from 2,  $g$ an endomorphism of
$g$ an endomorphism of 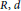 $R,\,d$ a Jordan semiderivation associated with
$R,\,d$ a Jordan semiderivation associated with  $g,\,F$ a generalized Jordan semiderivation associated with
$g,\,F$ a generalized Jordan semiderivation associated with  $d$ and
$d$ and  $g$, then
$g$, then  $F$ is a generalized semiderivation of
$F$ is a generalized semiderivation of  $R$ and
$R$ and  $d$ is a semiderivation of
$d$ is a semiderivation of  $R$. Moreover, if
$R$. Moreover, if  $R$ is commutative, then
$R$ is commutative, then  $F\,=\,d$.
$F\,=\,d$.