Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-28T03:26:15.240Z Has data issue: false hasContentIssue false
  • English
  • Français

Indecomposable Higher Chow Cycles

Published online by Cambridge University Press:  20 November 2018

Kenichiro Kimura
Kenichiro Kimura*
Affiliation:
Institute of Mathematics, University of Tsukuba, Tsukuba, 305-8571, Japan e-mail: kimurak@tsukuba.ac.jp
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

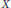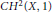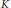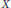Let $X$ be a projective smooth variety over a field $k$. In the first part we show that an indecomposable element in $C{{H}^{2}}\left( X,\,1 \right)$ can be lifted to an indecomposable element in $C{{H}^{3}}\left( {{X}_{K}},\,2 \right)$ where $K$ is the function field of 1 variable over $k$. We also show that if $X$ is the self-product of an elliptic curve over 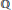$\mathbb{Q}$ then the 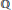$\mathbb{Q}$-vector space of indecomposable cycles 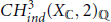$CH_{ind}^{3}{{\left( {{X}_{\mathbb{C}}},\,2 \right)}_{\mathbb{Q}}}$ is infinite dimensional.

In the second part we give a new definition of the group of indecomposable cycles of 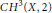$C{{H}^{3}}\left( X,\,2 \right)$ and give an example of non-torsion cycle in this group.

Keywords

14C2519D45
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 48 , Issue 2 , 01 June 2005 , pp. 237 - 243
Copyright
Copyright © Canadian Mathematical Society 2005

References

[AMS] del Angel, P. L. and Müller-Stach, S., The transcendental part of the regulator map for K 1 on a mirror family of K3-surfaces. Duke Math. J. 112(2002), 581–598.Google Scholar
[Be] Beilinson, A. A., Higher regulators and values of L-functions. J. Soviet Math. 30(1985), 2036–2070.Google Scholar
[Be2] Beilinson, A. A., Higher regulators of modular curves. Contemp. Math. 55(1986), 1–34.Google Scholar
[Bl] Bloch, S., Lectures on algebraic cycles. Duke University Mathematics Series, IV, Duke University Mathematics Department, Durham, NC, 1980.Google Scholar
[Bl2] Bloch, S., Algebraic cycles and higher K-theory. Adv. in Math. 61(1986), 267–304.Google Scholar
[BlKa] Bloch, S. and Kato, K., L-functions and Tamagawa numbers of motives. Progr. Math. 86(1990), 333–400.Google Scholar
[DwFr] Dwyer, W. G. and Friedlander, E. M., Algebraic and étale K-theory. Trans. Amer. Math. Soc. 292(1985), 247–280.Google Scholar
[EV] Esnault, H. and Viehweg, E., Deligne-Beilinson cohomology. Perspect. Math. 4(1988), 43–91.Google Scholar
[Fl] Flach, M., A finiteness theorem for the symmetric square of an elliptic curve. Invent. Math. 109(1992), 307–327.Google Scholar
[Gi] Gillet, H., Riemann-Roch theorems for higher algebraic K-theory. Adv. in Math. 40(1981), 203–289.Google Scholar
[GL] Gordon, B. and Lewis, J., Indecomposable higher Chow cycles on products of elliptic curves. J. Algebraic Geom. 8(1999), 543–567.Google Scholar
[Ja] Jannsen, U., Continuous étale cohomology. Math. Ann. 288(1988), 207–245.Google Scholar
[Ja2] Jannsen, U., Mixed motives and algebraic K-theory. Lecture Notes in Mathematics, 1400, Springer-Verlag, Berlin, 1990.Google Scholar
[Ki] Kimura, K., K 2 of a Fermat quotient and the value of its L-function. K-Theory 10(1996), 73–82.Google Scholar
[Ki2] Kimura, K., Elliptic units in K 2 . J. Number Theory 101(2003), 1–12.Google Scholar
[Ki3] Kimura, K., On K 1 of a self-product of a curve. Math. Z. 245(2003), 93–96.Google Scholar
[MeS] Merkurjev, A. and Suslin, A. A., The group K 3 for a field. Math. USSR Izvestija 36(1991), 541–565.Google Scholar
[Mi] Mildenhall, S., Cycles in a product of elliptic curves, and a group analogous to the class group. Duke Math. J. 67(1992), 387–406.Google Scholar
[MS] Müller-Stach, S., Algebraic cycle complexes. NATO Adv. Sci. Inst. Ser. C 548(2000), 285–305.Google Scholar
[MS2] Müller-Stach, S., Constructing indecomposable motivic cohomology classes on algebraic surfaces. J. Algebraic Geom. 6(1997), 513–543.Google Scholar
[MSC] Müller-Stach, S., Saito, S., and Collino, A., On K 1 and K 2 of algebraic surfaces. K-theory 30(2003), 37–69.Google Scholar
[Sa] Saito, M., On Mildenhall's theorem. preprint, AG/0106124, 2001 Google Scholar
[Sc] Schneider, P., Einladung zur Arbeitsgemeinschaft in Oberwolfach über “Die Beilingson-Vermutung”. Perspect. Math. 4(1988), 1–35 Google Scholar
[So1] Soulé, C., Operations on étale K-theory. Applications. Lecture Notes in Math., 966, Springer, Boston, 1982, pp. 271–303.Google Scholar
[So2] Soulé, C., p-adic K-theory of elliptic curves. Duke Math. J. 54(1987), 249–269.Google Scholar
[Sp] Spiess, M., On indecomposable elements of K 1 of a product of elliptic curves. K-Theory 17(1999), 363–383.Google Scholar